4. Who is Right?

• Tape measure for determining height
• Who is right? worksheet - At level: docx PDF
• Who is right? worksheet - Enable: docx PDF
• Who is right? worksheet - Extend: docx PDF
• Guinness World Records

How tall am I?

Ask students to guess your height. Students will provide a range of estimates; some will be close; others will be way off. Record all the suggestions on the board. 

Have students arrange the predictions from smallest to largest. Next, help them to narrow down their predictions. You might invite a student in the class to measure an item in the classroom which is similar to your height, or which can be used to compare you against. 

Prior to the lesson know the rough measurement of one or two items in you class so that you can ‘randomly’ select them off the top of your head. 

Ask students to compare you to this height:

Am I taller or shorter than the chosen item?

Am I double its height?

How much more or less, as a fraction, of the item am I? 

To help narrow the height down and to provide students with a fun fact announce to the class that the world record for the tallest person ever is held by Robert Wadlow and that his height was 272 cm. Use a measuring tape to show the students how tall this is. Ask the students:

Do you think I am more or less than half his height? How much shorter am I than he?

Here is a link to the Guinness Book of World Records. It has several pictures of Robert. 

Enable students by taking a 1 metre ruler and placing it against you. Ask students to determine if your height is double the 1 metre ruler or closer to another half of the ruler instead. 

Extend students by sharing your height with them and asking them to compare you with Robert Wadlow. How much taller is he? Can they calculate a percentage difference between you and his height? Encourage students to share their height using a range of different units. Can they describe their height in millimetres, centimetres, and metres? Can they describe their height in feet and inches? 

Working in pairs, students are presented with an area problem and four possible solutions. Students determine which of the four solutions are correct and then they describe how each person arrived at their solution. 

There are three levels of questions and possible solutions that students can choose to work on. Access downloadable versions of each sheet from the Materials and texts section above. 

Introduce to students the task using the following instructions: 

"My friend teaches at another school close by. She teaches English and Humanities but the other day she had to help and take a maths class. She gave the class a couple of area problems and received a range of different answers, and she is unsure which students are correct. I told her I would ask my maths class to help.  

Today I want you to select one of the three worksheets and determine which of the four solutions are correct and then describe in words how each person arrived at their solution. I want you to work with someone else who has also picked the same sheet as you. To help you select the worksheet which is just right for you I suggest you pick:

- Worksheet 1 if you are beginning to understand how to calculate area of squares and rectangles

- Worksheet 2 if you know how to calculate the area of squares and rectangles and are just beginning to understand how to calculate the area of a triangle

- Worksheet 3 if you can confidently calculate the area of squares, rectangles, and triangles."

Enable students should select worksheet 1. 

Extend students select worksheet 3. 

You may decide it is necessary to create additional sheets for your class, depending on the learning needs of your students. 

Importantly, this activity demonstrates that there is more than one way of arriving at a correct solution. Facilitate a discussion with students about why we use rules in mathematics. Prompt students to see that measurement and geometry mathematics have many conventions which have been agreed upon by mathematicians, however these are merely conventions and there is nothing stopping students from creating their own conventions based on their conceptual understanding of area. 

There are a small number of intentional mistakes on these worksheets. This will allow you to identify if the students look carefully through the students’ solutions and analyse their responses, as opposed to just answering the question themselves. 

Areas for further exploration

Area of a circle - adapted from a maths300 lesson (reproduced with permission). You will need to create an account to log in to maths300 to view the original problem.

Students use their knowledge of the area of a rectangle to devise their own formula for the area of a circle. This activity provides opportunities for students to develop conceptual understanding of the area of a circle.

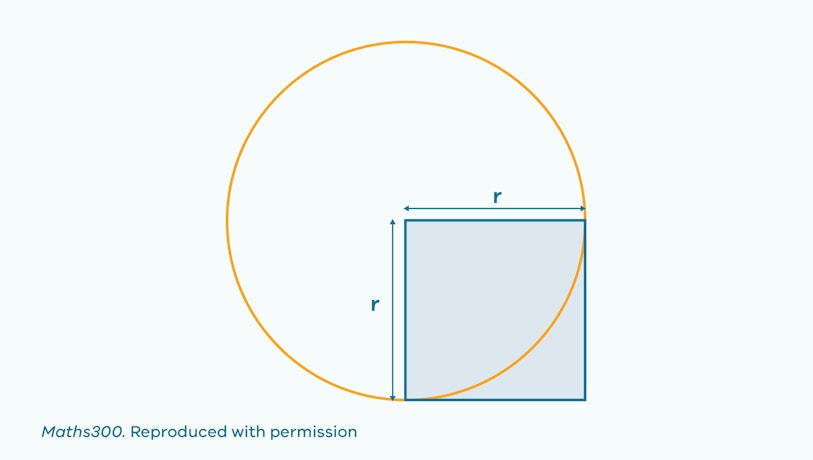
This activity supports students to use something they know ‘the area of a square’ and apply this knowledge to create new knowledge ‘the area of a circle’. Students identify the radius and then draw a square using this radius. From this they estimate how many of these squares would fit inside the circle. This will lead students to discover that there are less than 4 squares but more than 3 within the circle. Encourage students to see the link between this number and the formula for area of a circle:

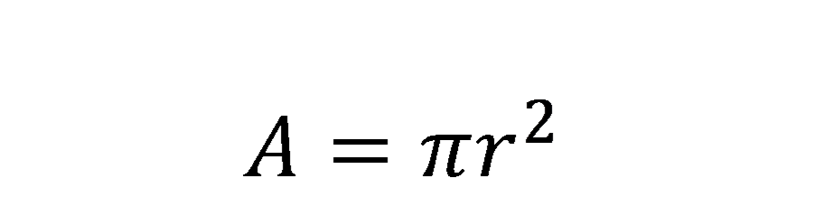
When formally assessing students at the conclusion of this stage you may decide to create a range of questions like those contained within the explore activity.

Include questions have been solved using common misconceptions and common errors for example when calculating area have some works samples which confuse perimeter and area or when calculating the circumference have some work samples which confuse radius and diameter.

Offer students a selection of questions to solve themselves and some which require them to explain how each student came up with their solution.

Have students describe the processes used and identify the mistakes that may have been made. You might consider using some de-identified student work samples from previous years to stimulate a discussion on how a problem has been approached and solved. 

Black Douglas Professional Education Services, 2017. Mathematics Task Centres: What's It Worth?. [Online]
Available at: http://www.mathematicscentre.com/taskcentre/075worth.htm
[Accessed 22 May 2020].

Guinness World Records Limited , n.d. Robert Wadlow: Tallest man ever. [Online]
Available at: https://www.guinnessworldrecords.com/records/hall-of-fame/robert-wadlow-tallest-man-ever
[Accessed 15 March 2022].