2. Uncle Jack's Land

• square centimetre grid paper or square counters: docx PDF

Students work in pairs to solve the following problem. 

"Four friends decide to compare the distance they each walk in one week. The only problem is at the beginning of the week they forgot to agree on the unit of measure they would use to record their walk. 

The four friends’ distances are:

• 380 metres
• 0.50 kilometres
• 5000 centimetres
• 80 metres + 0.3 kilometres (this friend used a different unit for each walk they took)

Who walked the farthest?"

Encourage students to rank them in order from farthest to shortest distance.

Once tasked with this question some students will ask which unit they should convert each measurement to. Use this opportunity to remind students that when representing a measurement, it is important to pick the most appropriate unit. Picking the most appropriate unit depends on the size of the amount to be measured, the attribute to be measured and the degree of accuracy required. 

Ask students if it matters which unit of measure is chosen. Do they recognise that as this task requires them to directly compare each measurement, it should not matter which unit they choose?

Enable students by asking them to write down all the different measurements which are equivalent to 1 metre. Encourage them to use this length as a starting base to compare all the units described above. 

• 1 metre = 100 centimetres 
• 1 metre = 0.1 kilometres 

Extend students by pre-preparing additional distances to be compared. Depending on the knowledge and skills of your students you might include non-metric units. 

This activity is reproduced with permission by Rob Vingerhoets.

Prior to commencing this activity, ensure that students know the difference between perimeter and area. 

Introduce to students the following scenario:

My Uncle Jack has just passed away and, in his will, he has left me 60 square metres of land. The block of land is in a remote area of Queensland, so I have not seen it. Last night I started to wonder what it might look like. 

Ask the class to come up some ideas about what the land might look like. Encourage students to be creative - L shapes, triangular blocks of land, hexagonal blocks, triangular and even a circle.

Depending on the level of experience and competence of students in your classroom, set an expectation for the number of designs that each pair of students will come up with. You might decide to make different specifications for different groups of students.

Enable students by reducing the number of required designs or encouraging them to use simple rectangular shapes. Provide concrete material such as Unifix blocks, square tiles or grid paper that students can use to model their designs. Square tiles can be used to support students to draw out each block of land design. This strategy supports students to develop their multiplicative thinking and their understanding of area. Students draw out each land design and describe the array (area model) by describing the number of rows and the number in each row. Demonstrate that like an array, the number of rows is multiplied by the number in each row. For example, if there are 12 rows, and each row has five squares, 12 multiplied by five gives an area of 60 square units. 

The use of grid paper will bring up the concept of scale factor and enable students to see that each square centimetre is equivalent to a square metre. Grid paper is available to download from the Materials and texts section. 

Extend students by requiring them to design at least two shapes that are composite or non-rectangular shapes. Ask students to determine which block of land is the cheapest to fence. Inform students that in Queensland there is a law that if any block of land exceeds 59 square metres it must be fenced. If fencing costs $15 per metre, which design is the cheapest to fence?

At the conclusion of the activity students share their designs with others in the class. Ask the class if other groups had similar designs to theirs. How could this be possible if each group came up with their own designs? Prompt students to see that there is a maximum number of combinations possible and therefore it is likely that a few others in the class will have a similar design. 

Areas for further exploration

Task 1. Exploring area and perimeter

Prepare either different size blocks of land or different examples of 60 m2 blocks of land. These blocks of land could be made up of composite shapes, so that students first need to partition the shape into the smaller parts. Provide a variety of different shapes with varying complexity to challenge the students in your class. Ask students to find the area and perimeter for each shape. Ask students to determine what shape block would be the most cost effective if they had to build a fence that cost $20 per metre length.  

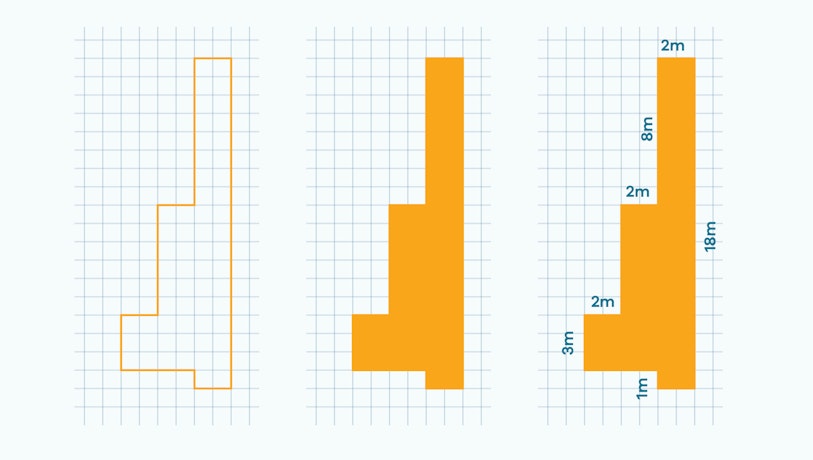
Task 2. Equable shapes

An equable shape is also known as a perfect shape. It is a two-dimensional shape whose area is equal to its perimeter. For example, a circle which has a radius of 2cm has both an area and a perimeter of 12.56637.

In this activity, students further explore the relationship between area and perimeter. This is a very challenging activity that may not be suitable for all students in your class. In the beginning students use trial and error or a spreadsheet to find their answer, however this activity encourages students to prove their answer using sophisticated algebra and equation solving including Diophantine equations. 

There are an infinite number of equable shapes however, if you restrict the sides of the shape to an integer, then the number of possible shapes decreases greatly. There are only two equable triangles, three equable scalene triangles and two equable right-angled triangles. 

This stage introduces students to conversion of units and area. At the conclusion of this stage, consider setting a range of problem-solving tasks for students to answer. You might decide to set area problems with a different unit so that students must first convert before solving the area equation.

When writing area-based questions, please note that questions which ask students to calculate the area of a given shape are less challenging than those which ask students to prove a solution is correct or which require students to ‘work backwards’ to come up with the possible dimensions. 

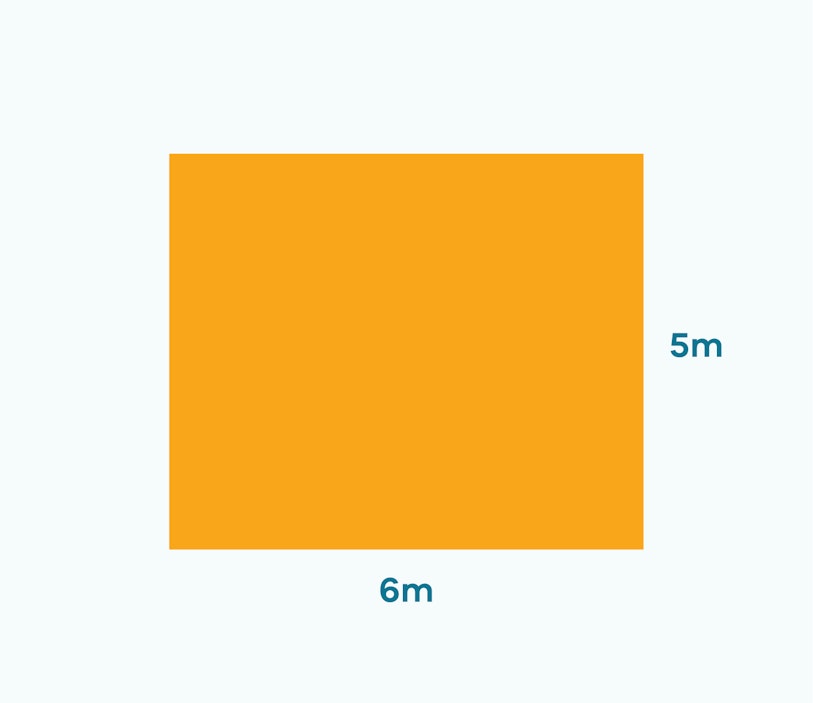
For example, calculate the area of the following shape:

You may decide to add algebra into your area questions to further challenge your students. For example:

• A plot of land is to be planted with lawn.
• Write an expression for the area of the lawn.
• How much lawn is to be planted if x is 5 metres?

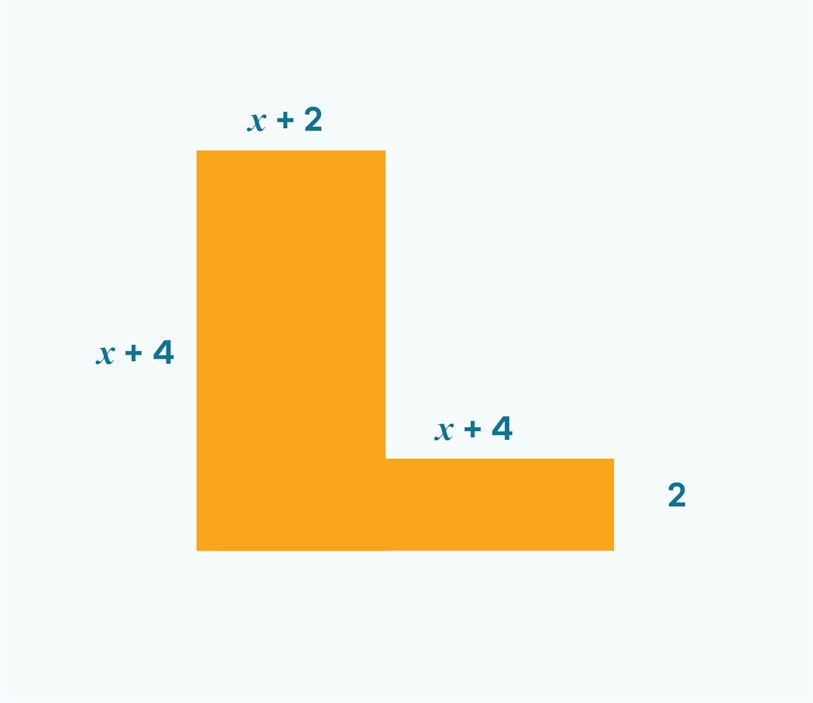
When assessing your students using the above consider the following: 

• Can the student convert between different units of measure?
• Does the student know the difference between perimeter and area?
• Can the student articulate how to calculate the area of rectangular and simple composite shapes?
• Can the student provide a range of reasonable responses when given the area, and asked to determine what the length of dimensions might be?

Ruthven, B., 2016. Equable Shapes. Vinculum: Mathematics Association of Victoria, 53(1), p. 10.

Vingerhoets, R., n.d. Maths Doesn't Have to Hurt. [Online]
Available at: http://robvingerhoets.com.au/maths/
[Accessed 15 March 2022].

Vingerhoets, R., n.d. UNCLE JACK’S LAND. [Online]
Available at: http://robvingerhoets.com.au/maths/wp-content/uploads/2017/11/Uncle-Jacks-Land-M-5-6.pdf
[Accessed 15 March 2022].