1. Fractions as Equal Parts of a Whole

• Pattern blocks
• Print outs of the diagrams used to partition
• Tangram shapes: docx PDF

Display a large image of a shape made from pattern blocks. An example of a triangle is provided here.

Ask students to refer to the shape displayed and identify as many fractions as they can if the entire triangular shape represents one whole.

Alternatively, pose a question such as:

'Using only the small triangles and trapeziums shown in the image, how could you represent ⁴/₉?'

Provide students with pattern blocks or other concrete models that they can manipulate. Pattern blocks will allow students to either split the trapezium into triangles or make trapeziums from the triangles.

Do not offer any other instructions or tell students what they need to do. Allow students time to come up with their own responses based on their understandings.

Ask students to draw and label their responses and invite them to discuss their findings.

To assist with facilitating the discussion, the following prompts may be helpful:

How many triangles can you see in this shape?

How many triangles could be used to form this shape? How many trapeziums could be used?

How many equal parts can the shape be divided into? The emphasis in this question is on the word equal.

Can the shape be divided into a different number of equal parts? Using the blocks shown, the triangle can be divided into 9 equal triangles or three equal trapeziums.

If the entire triangular shape represents one whole, how many different fractions of the whole can you make?

Extend students by posing a more complex problem such as:

If the entire triangular shape has a value of three, what would be the value of one small triangle? What would be the value of one trapezium?

Monitor student responses and address any misconceptions that indicate students do not understand the relationship between the denominator and the parts of the whole being of equal size. For example, students who notice that the shape consists of five parts may incorrectly conclude that:

• three triangles form ³/₅ of the whole shape, or
• one trapezium represents ¹/₅ of the whole shape.

Ask students to use the materials to check their original responses. Then ask students to recreate the entire shape using only small triangles, or only the trapeziums. Guide students to the understanding that:

• one trapezium is ¹/₃ of the entire shape, and
• one triangle is ¹/₉ of the entire shape.

If students have not understood how these fractions were determined, demonstrate how to use the materials to partition the shape to show equal parts.

Enable students by providing a simpler diagram such as the one shown here, which shows that the whole consists of three trapeziums of equal size. When students are confident with this type of shape, they can progress to more complex shapes.

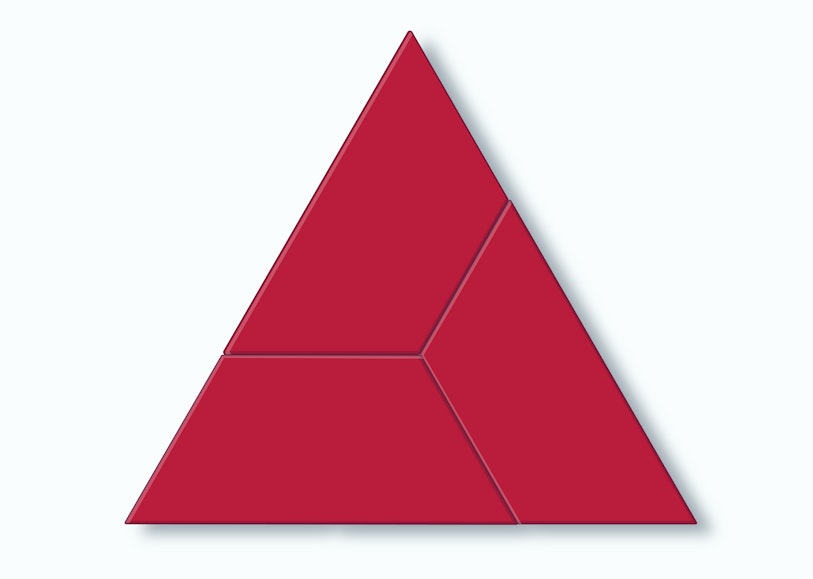
Extend students by asking them to investigate different types of problems related to the original pattern block diagram. For example, if the value of the entire shape is one half, what is the value of the trapezium? If the value of the small triangle is two thirds, what is the value of the entire shape?

The following activities provide opportunities for students to develop their understanding of fractions as equal parts of the whole.

1. Tangram puzzle

Display an image of a tangram.

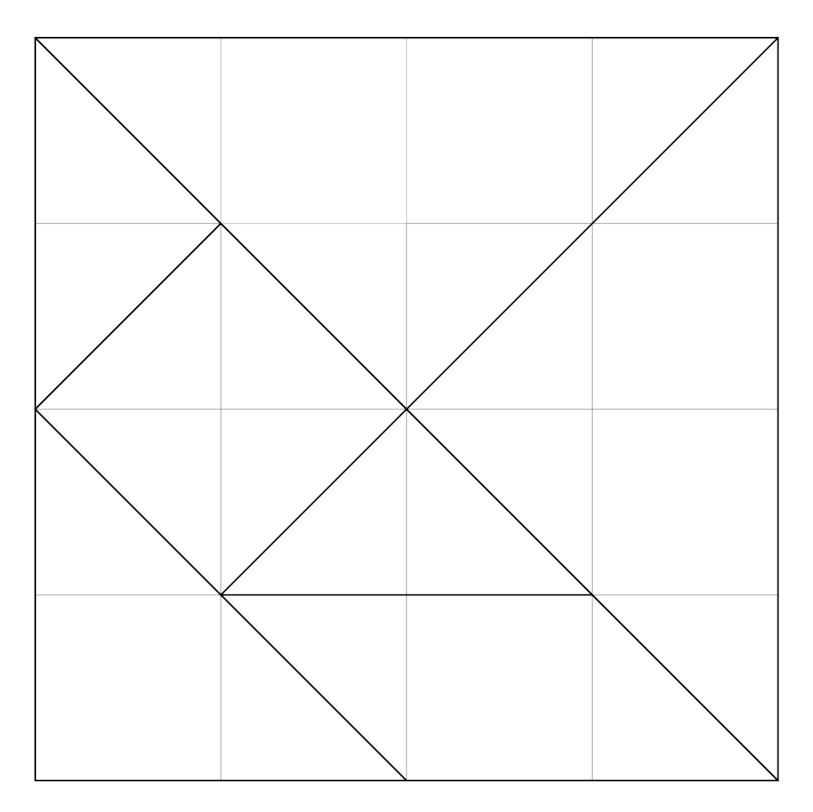
Provide manipulatives such as pattern blocks for students to recreate this shape, or provide multiple printed versions that students can cut up or draw on as required. 

Present students with this problem:                         

If this entire square represents one whole, how many ways can you represent one quarter?

Ask students to draw their representations and explain their reasoning. Possible discussion prompts include:

• What shapes make up the tangram?
• What fraction of the whole do each of the smaller shapes represent?

Enable students by providing a tangram where the partitions are visible. Ask students to consider the relationship between the different shapes that make up the tangram.

Extend students by changing the value of the tangram. For example, if the tangram had the value of 4, what would the value of each shape be?

2. Entangle the Rectangle

In this problem, one large rectangle is divided up into smaller shapes which are numbered and different coloured.

Ask students to determine the fractional value of each numbered shape within the larger rectangle, if the larger rectangle represents the whole. Ask students to share their thinking in pairs or small groups. Monitor student progress and ask prompting questions as needed. Sample prompts include:

• What is the biggest shape? What fraction of the whole is this shape?
• What is the smallest shape? What fraction of the whole is this shape?              

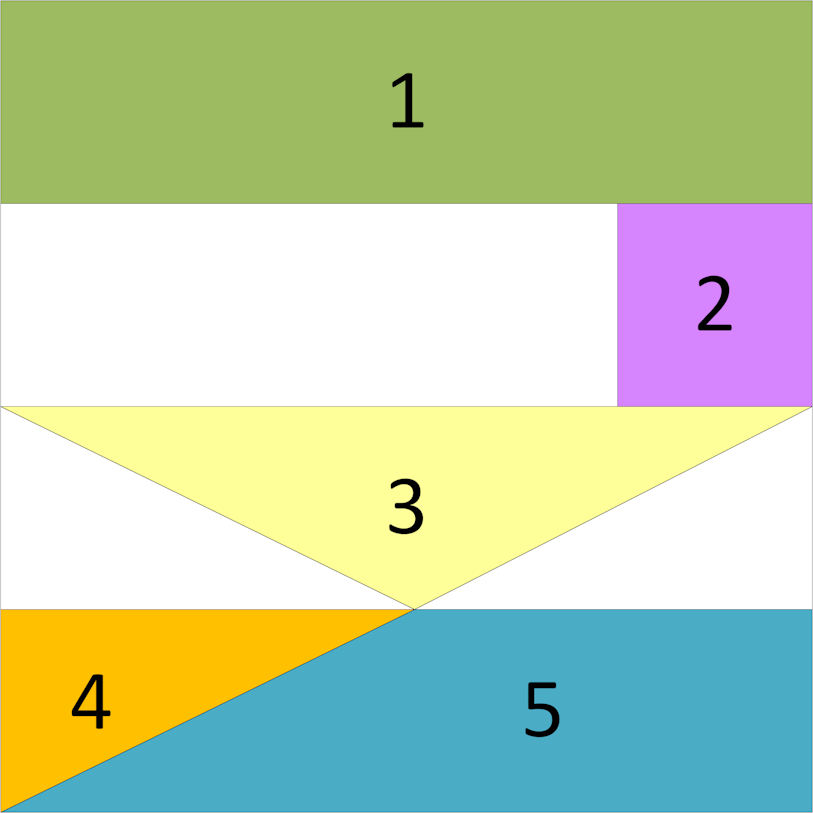
Enable students by providing the shapes on grid paper or by simplifying the number of shapes and the complexity.

Extend students by inviting them to create their own shape and work out the fractional parts. They could then swap their shapes with a partner (or multiple partners) and repeat the task using their partner’s shape.

This sequence has used an ‘area model’ to explore the concept of fractions as equal parts of a whole. Once students have developed their conceptual understanding of this fundamental idea of fractions, they can work on building their skill and understanding of other key ideas for fractions.

Check for student understanding of the concept by providing a different model that students can use to determine fractions of a whole. Use diagrams and models that are not partitioned to promote deeper thinking and problem-solving strategies.

For example, you could use a ‘length model’, by drawing a number line from 0 to 1.

Tell a story that permits you to ask fraction-related questions. For example:

'Jai rides his bike to school every day. On his way he makes several stops. First, he stops at his friend, Bruno’s house. Then they always stop and pat Patch, the friendly neighbourhood dog. One Tuesday, Jai was nearly at school when he got a flat tyre. If the following diagram represents Jai’s trip, what fraction could we use to represent how far he had ridden by each stop?'

Note: 0 represents Jai’s house and 1 represents the school.

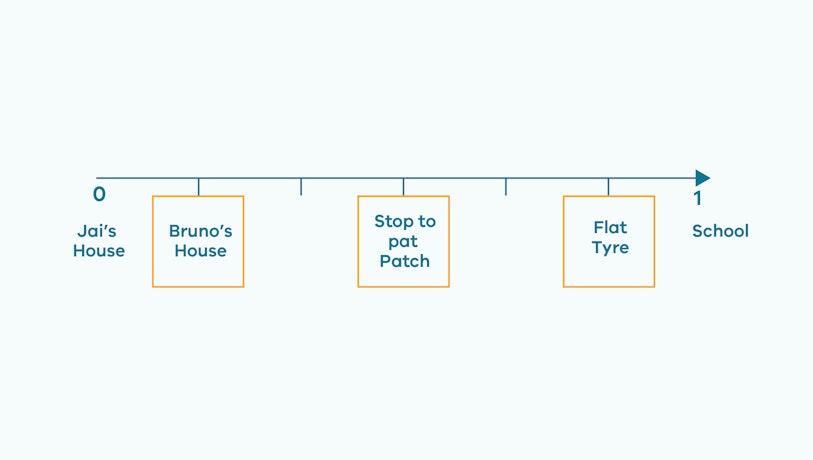
Encourage students to draw on their knowledge of fractions as equal parts of a whole to estimate the fraction at each stop. How could they divide the line to create equal parts?

Provide a collection of shapes divided into four parts and ask students to identify which are correctly divided into quarters (that is, four equal parts). Students could also explain their reasoning for each of their responses. Their responses could be used to help assess their understanding.

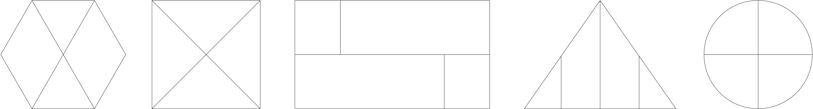
This task could be adapted to include other fraction names such as thirds or sixths.

Activity Village, 2019. Tangrams. [Online]
Available at: www.activityvillage.co.uk/tangrams
[Accessed 15 March 2022].

funmaths.com, 2011. Make a Tangram Puzzle. [Online]
Available at: www.funmaths.com/games_puzzles/downloads/tangram_template.pdf
[Accessed 15 March 2022].

Sullivan, P., 2017. Challenging Mathematical Tasks. South Melbourne: Oxford University Press.