Suggested Learning Intentions
- To understand fractions result from division
- To compare fractions, noting how the numerator and denominator affect the size of a fraction
Sample Success Criteria
- I can identify fractions that have different names but are the same size
- I can identify which fraction is larger or smaller based on the name of the parts and how many of these parts there are
- I can justify my thinking and solutions using manipulatives or other representations
This stage has been inspired by the Chocolate cake problem on Maths300, and is reproduced with permission. Access to the problem on Maths300 requires a subscription.
Introduce the concept of fractions with a story that prompts a problem-solving task. For example:
'The Sweet Treats cake shop makes the best chocolate cake in town. It is so popular that they don’t have time to cut it up and it can only be bought unsliced in rectangular trays. If your family bought a tray, how could it be shared so that everybody in your family got exactly the same amount?'

Provide students with a sheet of paper, or other materials, to represent the cake.
Ask students to determine how they would divide the cake equally amongst their family and record this as a fraction. Ask students to also consider their broader family size (for example, aunts, uncles, and cousins), particularly for students whose immediate family size will make this task simpler.
Allow students to choose how to show this (e.g., to fold and/or cut the paper, draw their responses or use rubber bands to divide a concrete rectangular shape). Using concrete materials that students can manipulate will help emphasise the idea of a fraction as equal parts of a whole.
Encourage students to work collaboratively with a partner who has a different number of family members. This provides opportunities for students to find different-sized fractions, such as thirds, fifths etc.
As students work, ask questions to prompt their thinking, such as:
- What might you need to consider before you divide the cake into equal parts?
- Who in our class might get the most/least cake when it is shared with their family? Explain your thinking.
- What are some of the strategies you could use?
- How could you represent the amount of cake you would receive as a fraction?
Invite students to share their work with the class. Ask them to explain the method they used and the fractions they recorded.
Display samples of the students’ responses. Discuss their numerical representations of fractions and encourage students to identify the link between the size of the denominator and the size of the fraction. Question prompts include:
- Why might it be important to divide the cake into equal parts?
- What does the top number (the numerator) represent?
- What does the bottom number (the denominator) represent?
- How does the size of the fraction change as the numerator gets larger? Is this always the case?
- If we needed to divide the cake between 6 people rather than four, how would that change the amount of cake each person would receive, and the way we record that as a fraction?
When explaining the numerator and denominator of a fraction, discuss the denominator in terms of the name or size of the part. For example, fifths have this name because 5 equal parts fill a whole. The numerator is the number of parts of that name or size (Clark, Roche, Mitchell 2011). Explaining the denominator and numerator in these terms may help students make sense for improper fractions when they encounter them.
It is important for students to understand the fraction as a part-whole relationship where each part is equal in size.
Pose problems for the students to solve. For example:
'I invited two friends to lunch, so I made three salad sandwiches. Two extra friends arrived unexpectedly so I only had three salad sandwiches to share between five people.'
Ask students:
- How could I divide the three sandwiches equally between the five of us?
- What fraction of a whole sandwich will each person receive?”
Encourage students to share their thinking and model their problem-solving strategies to their peers.
Provide a range of concrete materials for students to work to model the problem.
Extend students by asking them to consider what fraction of the 'three sandwiches' each person will receive, if the three sandwiches are considered as the whole. It is important for students to consider that the size of the fraction is always related to the definition of the whole. In this case, the fraction ³/₅ can be used to represent how much each person will receive, if the whole is considered to be one sandwich. However, the fraction ¹/₅ can be used if the whole is considered to be three sandwiches.
Introduce a second problem to the class:
'Imagine that you all love chocolate cake and want to have as much of the cake as possible. I have bought six trays of chocolate cake from the Sweet Treat bakery and have placed them on three tables. I put one cake on table 1, two cakes on table 2 and three cakes on table 3. I am going to invite ten friends over for a party and we will share the cakes.'
Ask ten students to volunteer as your ‘guests’. Explain to the class that the guests will be invited to sit at a table one at a time. When selecting which table to sit at, they may ask themselves this question:
'If the cake on the table I sit at is to be shared equally when I sit down, which table would be the best to sit at?'
A4 sheets of paper or A4 paper copies of a rectangular chocolate cake image can be used to represent the six cakes. The number of students and the number of cakes could be changed to any value.
Before the ten students select their tables, explain to the remaining students what they are required to do.
1. Predict which table each guest will sit when it is their turn.
2. Record the changing amount of how much cake each student will get as each new student selects a table to sit at. For example, if student 1 sits at table 1, they will get the whole cake. Later, if student number 4 sits at table 1, they will each get ¹/₂. Then if student 10 also sits at this table each student will now get ¹/₃ of the cake. An activity sheet to record results is available in the Materials and texts section above). Students could also use diagrams or concrete materials such as counters to represent their understanding.
3. Work out how much cake each student gets. Who gets the most cake?
Provide enough paper for the remaining students to explore their ideas and thinking.
When the party is ready to begin, ask the ten students, one at a time, to decide which table they wish to sit at. Explain that students cannot change tables once they are seated, and will need to wait until all ten students have taken a seat before they can share the cake on their table.
As each volunteer has their turn, allow them enough time to think about which table would be best to sit at to ensure they get the most chocolate cake.
Once all ten students are seated, ask them to work collaboratively in their table groups to determine exactly what fraction of cake each of them will get at their table. Allow students to use any strategy they wish to work this out, including measuring or folding the paper.
The observing students could also form small groups to calculate the fraction of cake each table group would receive.
Ask one student from each group to report back to the class about the strategies they used to work out how much cake they each get. For example, at table 3 they may have to share three cakes amongst four students. The more students that are seated at the table, the more complex it becomes to work out how much cake each person will get.
Once each table has come up with their answers, ask if any of the guests would like to change tables based on the fraction of cake they will get at their table. Allow guests to move one at a time, and after each move, ask students to recalculate the fraction of cake that they will receive at their table. If a guest doesn’t allow for these changes, they may receive less cake than they started with!
Use the results from the three tables to compare fractions with the class. For example, suppose on table 2 each person receives ²/₃ of a cake and on table 3 each person receives ³/₅ of a cake. Ask students to determine which fraction is bigger.
This may prompt a whole class discussion of the comparison of fractions and strategies to determine which one is the larger fraction. One strategy for comparing size is to compare or ‘benchmark’ a fraction to another well-known fraction, such as a half, or a whole number such as 0 or 1.
'Chocolate Cake' exposes students to a range of mathematical skills and concepts which you may wish to review and consolidate with students. Consider the progress made by students when determining which skills and concepts you select to review.
1. Reflection on the task
Reflect on the task with the class. Possible discussion prompts include:
- Compare the fraction of cake per person on each table with the fraction each person would get if the six cakes were shared equally between the ten guests.
- If each table had only one cake, how could the three cakes be divided so that all ten students got exactly the same amount?
- If the six cakes were shared equally amongst your family, how much would each person get?
- How much cake would each person receive if you joined them at the first table? How about on the second table? ... third table?
- Were the six cakes shared equally? Could we arrange the table groups so that all ten students received the same fraction of the cake?
2. Fractions as division and equal parts of a whole.
Revisit the success criteria of this stage:
- I can identify fractions that have different names but are the same size.
- I can identify which fraction is larger or smaller based on the name of the parts and how many of these parts there are.
Provide a question prompt that allows students to demonstrate their understandings of these concepts. For example:
'My friend picked up 3 pizzas for dinner to be shared equally amongst 4 people. Unfortunately, the pizza shop did not cut up the pizzas for us. We all love pizza, so we each want to get an even share. How do we much pizza does each person get?'
Ask students to demonstrate how they would cut the pizzas into four equal shares, and to identify the fraction that each person would receive. They may use any materials they wish to assist them.
Observe the methods used by students to answer this problem. Encourage students to select a strategy and draw or create a model to illustrate their answer.
For example, students could draw 3 circles and divide each one into quarters. Two slightly different representations are shown.
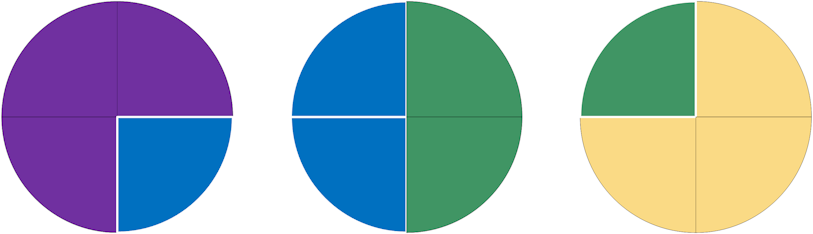
OR
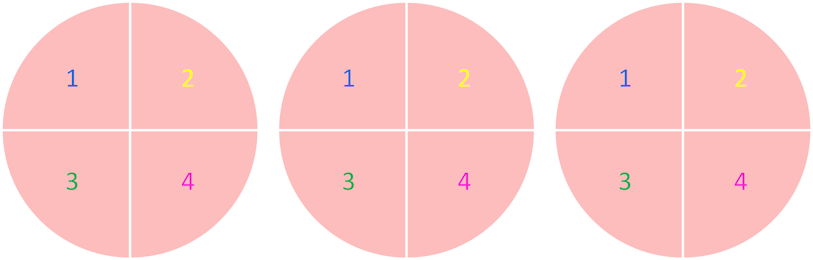
Enable students to divide the pizzas into equal fractions by altering the denominator to create a simpler fraction. For example, they could share 3 pizzas between 6 people.
Extend students by asking them to also record the fraction each person will receive of the three pizzas (that is, where the three pizzas represent the whole). This question will reinforce the understanding of the relationship between the denominator and the whole. You could also make this activity more challenging by selecting different numbers. For example, to share five pizzas amongst eight people or eight pizzas amongst five people.
Ask students to share their work and discuss the strategies they used. Promote discussion of different strategies. Possible discussion prompts include:
- What helped you with your thinking?
- Were there any aspects of this question that you found challenging?
- How would the answer change if we referred to the three pizzas as one whole, rather than one pizza as a whole?
Collect student work to assess their understanding of fractions as division.
3. Estimating and comparing the size of fractions or decimals
Remind students of the sharing chocolate cake activity. Facilitate a discussion that compares fractions and focusses on the relationship between the denominator and the numerator. Ask who they thought had received the largest/smallest fraction of the chocolate cake and why this was the outcome.
Display a fraction wall and invite students to make statements using the language of 'greater than' and 'less than'. For example, one ninth is less than one seventh.
Suggested questions:
- What do you notice about the size of the unit fractions?
- Is one third greater than or less than one fifth?
- Is one third greater than or less than two fifths?
Provide a range of questions on the board for students to select from to complete an exit ticket. For example:
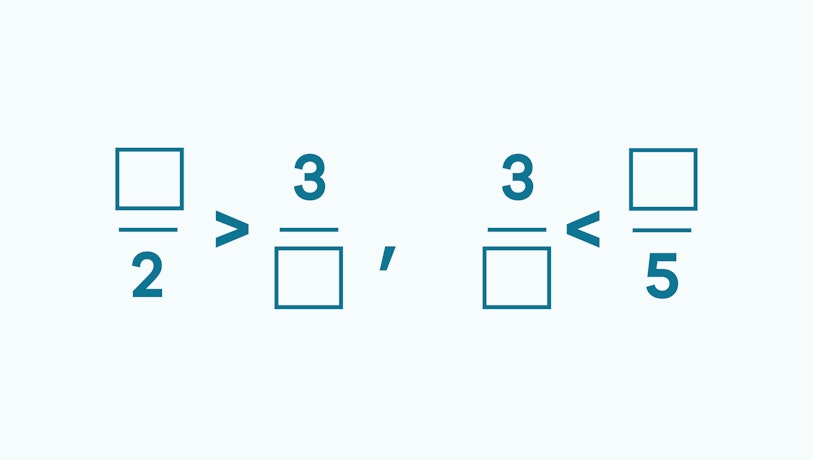
Students offer possible responses and explain their reasoning.
Collect student work and invite questions and comments about the task.
Australian Association of Mathematics Teachers, n.d. Chocolate Cake. [Online]
Available at: maths300.com/members/m300full/135lchoc.htm
[Accessed 15 March 2022; Note: access to this resource requires a maths300 subscription].
Visnos Mathematics, 2019. Fraction Wall. [Online]
Available at: www.visnos.com/demos/fraction-wall
[Accessed 15 March 2022].
Other stages
1. Fractions as Equal Parts of a Whole
EXPLORESuggested Learning Intentions
- To understand that fractions can represent equal parts of a whole
Sample Success Criteria
- I can accurately identify the name of each fraction part
- I can correctly name fractions using the number of equal parts needed to make up the whole
- I can explain and justify my solutions using a variety of manipulatives
3. Fractions to Decimals
EXPLORESuggested Learning Intentions
- To estimate and locate fractions and decimals on a number line
- To understand fractions and decimals as different representations of the same number
Sample Success Criteria
- I can locate simple fractions, improper fractions, and decimals on a number line
- I can represent fractions as decimals using different models: area models, number lines and set models
- I can demonstrate my understanding using a variety of manipulatives
4. Colour in Fractions
EXPLORESuggested Learning Intentions
- To understand two fractions can be the same size but have different names
Sample Success Criteria
- I can identify fractions that are the same size but have different names
- I can make fractions that are equal in size using different parts
- I can use manipulatives to model and explain my thinking and solutions
5. Colour in Decimats
EXPLORESuggested Learning Intentions
- To understand place value in decimals
- To understand how place value determines the size of a decimal
Sample Success Criteria
- I can accurately colour decimals on a decimat to demonstrate place value
- I can order decimals correctly on a number line
- I can use different manipulatives to model and explain my solutions and thinking