5. Interpreting Designs

• Plastic or wooden pattern blocks
• Kinder square paper
• Dot grid paper: docx PDF

Introduce the ‘Barrier Game’ to students, played in pairs:

• Students begin with a collection of the same pattern blocks in front of each of them and a barrier between them.
• One student creates a construction hidden from view and describes it to the other using geometric and location language.
• Their partner recreates the structure following the instructions.
• At the end of the game, the barrier is lifted to reveal and compare the constructions
• Swap roles and replay so that both students practice communicating using geometric and location language, and listening to instructions.

Play this game again but this time, instead of constructing structures, have one student use dot grid paper to draw a design and the other follows the instructions to recreate the drawing.

Alternatively, students could sit facing each other so that one student has their back towards the front of the class and the other student is facing the front. Display a drawing on the board so that the students facing the front can give instructions for their partners to draw.

Observe student's instructions to check that they are using formal geometric and location language to describe the position of the shapes clearly.

Extend students by asking them to draw shapes within a shape. For example:

These activities challenge students to use their understanding of geometry and visualisation skills to investigate spatial problems and aim to help students develop visual images of geometric shapes and objects. Although activities in the previous stages have involved using these processes, the following activities aim to develop student visualisation skills and spatial reasoning in other aspects.

1. Visualising symmetry

Introduce the context of the activity by explaining that sometimes, stencils are used to enable repeated patterns or shapes to be made with ease. Ask students to brainstorm examples of where stencils are used. For example, stencils can be used to help when painting border designs. Explain to students that in this activity, they are to imagine that they are creating stencils for symmetrical designs, which can be used to paint their wall decor in their house.

Show students an irregular polygon such as:

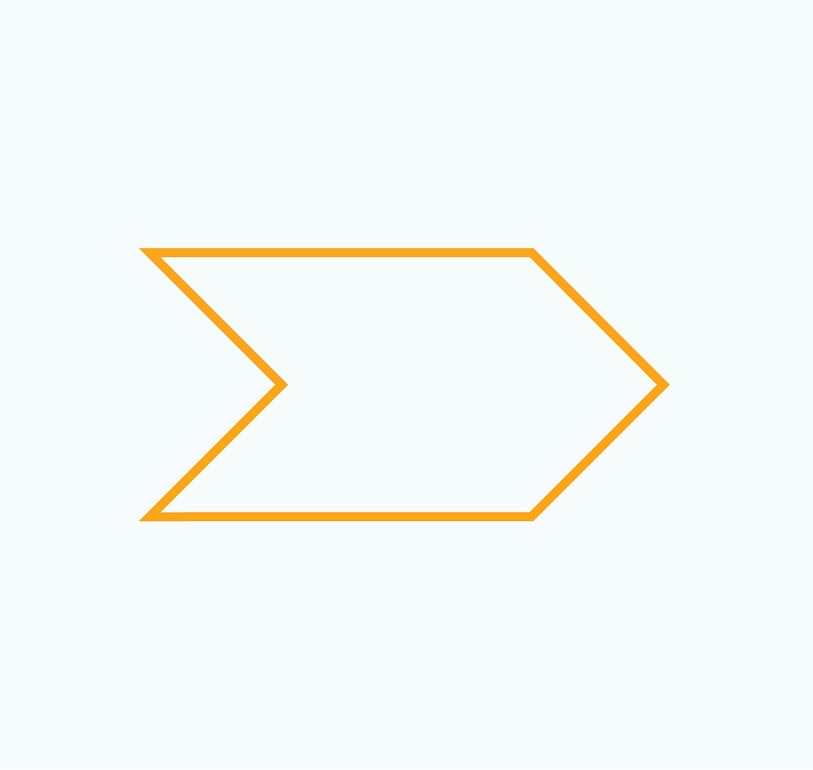
Give each student a kinder square. Ask them to fold and cut the paper in a way so that when it is unfolded, it will reveal the shape shown above. Do not tell students which way to fold the paper. Encourage students to work in pairs or small groups and discuss their strategies and predict the outcome before making the cuts to create the shape with a folded kinder square.

Students to share their solutions. Encourage students who did not succeed to talk about their thinking process when trying to cut out the shape and discuss what they may do differently next time. Ask students if they have changed their minds after seeing other students’ strategies.

Invite students to draw other polygons and to challenge their peers to reproduce the shape by folding and cutting kinder squares. Encourage students to explore folding paper horizontally, vertically, and diagonally and observe how changing the fold line can change the resulting pattern.

As a further challenge, show students a kinder square, fold it into half, making a rectangle. Then fold it into half again, making it into a smaller square. Cut out a triangle from the folded corner.

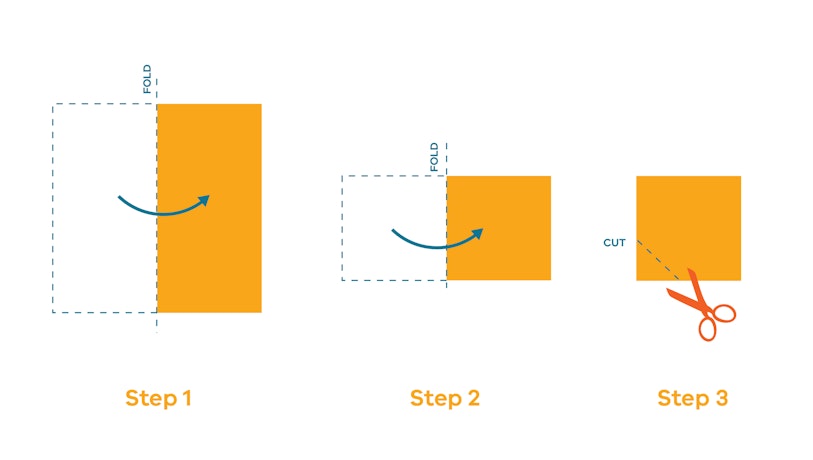
Ask students to collaborate in small groups to predict what shape will be created in the middle of the kinder square and what the kinder square will look like when unfolded. Encourage students in each group to discuss and justify their reasons for their predictions. Then, provide students with kinder squares to explore the solution and to check their predictions.

Repeat the process with other folds and cuts, such as:

Fold a kinder square into half diagonally, making a triangle. Fold into half again, making a smaller triangle. Make a cut on the ‘flap’ vertex, from one edge of the triangle to the other. Ask students to predict what the kinder square will look like when it is unfolded.

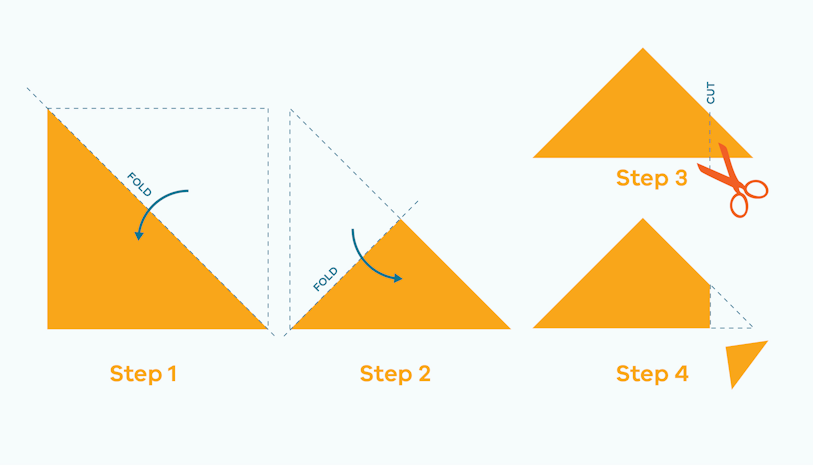
Enable students by reducing the number of folds of the kinder square, initially just folding once in half. Ask students to create different shapes by making cuts on the fold or flap edges. Challenge students to make a regular polygon by cutting from the fold edge. Once students become more confidence, encourage them to fold the kinder square twice and to predict the shapes that will be formed when they cut on the folds before opening the kinder square.

Extend students by asking them to predict the result from making multiple cuts, for example one from the folded edge and one from the flap edge. Allow students to explore what will happen if other shaped paper is used, such as an A4 piece of paper or a kinder circle.

2. Visualising transformation

Give each student 5 square tiles.

Explain to students that each square tile represents a modular shelf unit for their new home. It may be useful to show students images of modular units used as furniture so that students can understand the context of the activity.

Ask students to put the 5 square tiles together, each square touching at least one other square by sharing a full side. This will form a shelf design. If students start to stack the square tiles instead of placing them side by side, guide students to differentiate between the term ‘side’ and ‘face’.

Students explore the different modular shelf designs that they can make and record them on grid paper. Designs are the same if one can be rotated or reflected to match the other. How many different designs can be made?

As students are exploring their designs, ask students prompting questions such as:

• “How do you know that these two shapes are different?”
• “How do you know that you have found all possible solutions?”

When students think they have recorded all possibilities, have them compare their solutions with a peer. Create a class anchor chart showing the 12 different possible pentomino designs.

Enable students by reducing the number of square tiles for their design.

Extend students by asking them how would the number of possible solutions change when we use six tiles? What about seven tiles? Is there a pattern?

The designs created by the students, where five squares are combined so that each one shares at least one side, are known as pentominoes.

As a further extension, students can solve puzzles using the pentominoes that they have created.

Students could use the pentominoes function available on PolyPad or ask them to cut out the twelve pentomino shapes they found to use as pieces for the puzzles. For the context of creating interior designs, ask students to combine three different modular unit designs (or pentominoes) to fit a 5 x 3 square grid space.

Challenge students to explore other puzzles. For example:

• Use four different pieces to fit a 5 x 4 grid space.
• Use nine different pieces, except the L-shape, to create a giant L-shape
• Fit all twelve pentomino shapes in an 8 x 8 grid, so that there are four grid squares blank.

Invite students to share their solutions with others and explain the strategies they use. Ask students to identify the similarities and differences between their solutions and their peers.

Areas for further exploration

1. Visualising cubes

Students work with a partner to solve the Colourful Cubes problem from Nrich Maths. This activity gives students the opportunity to develop their visualisation skills by working out how many cubes of each colour is needed to make a 3 x 3 x 3 cube.

This stage of the sequence gives opportunities for students to develop their visualisation skills and geometric reasoning. Students should be able to create mental images of shapes formed from combining or splitting shapes as well as use their imagination to predict the results of manipulating and making transformations to shapes.

Formative assessment of student learning in this stage of the sequence could include discussions with students and encouraging them to justify their thinking. Ask students questions to prompt reflection, such as:

• 'What made you think you had to rotate the shape?'
• 'What would you have done differently?'
• 'What patterns are you noticing?'

As students are working in the activities throughout the stage, observe how students solve problems and ask them to explain what they have done.

Visualising symmetry

Check for students’ ability to create mental images and application of the concept of symmetry by asking them to create a given shape by folding and cutting kinder square paper. The focus is on students’ strategy to problem solve rather than whether students can correctly recreate the shape.

Show students a range of kinder squares or circles that have been folded, several cuts made and unfolded. Ask students to choose a design and challenge them to recreate the design by folding and cutting.

Booker, G., Bond, D., Sparrow, L. & Swan, P., 2014. Teaching Primary Mathematics. Melbourne: Pearson Australia.

BrainPOP, n.d. Blockly: Maze. [Online]
Available at: https://www.brainpop.com/games/blocklymaze/
[Accessed 15 March 2022].

Code.org, 2021. Course E. [Online]
Available at: https://studio.code.org/s/coursee-2021?redirect_warning=true
[Accessed 15 March 2022].

Glenrock Consulting, 2013. How Did They Make Ms. Pac-Man?. [Online]
Available at: https://robertkaplinsky.com/work/ms-pac-man/
[Accessed 15 March 2022].

Google, n.d. Google Maps. [Online]
Available at: https://www.google.com.au/maps
[Accessed 15 March 2022].

Google, n.d. My Maps. [Online]
Available at: https://www.google.com.au/maps/d/u/0/
[Accessed 15 March 2022].

Learning Resources and Technology Services, n.d. Square Dot Paper. [Online]
Available at: https://lrt.ednet.ns.ca/PD/BLM/pdf_files/dot_paper/sq_dot_1cm.pdf
[Accessed 15 March 2022].

Look Cool, 2014. Symmetry. [Online]
Available at: https://online.clickview.com.au/libraries/videos/9550830/symmetry
[Accessed 15 March 2022].

Math Learning Center, n.d. Geoboard. [Online]
Available at: https://apps.mathlearningcenter.org/geoboard/
[Accessed 15 March 2022].

Math Playground, n.d. Area Blocks. [Online]
Available at: https://www.mathplayground.com/area_blocks/index.htm
[Accessed 15 March 2022].

Mathigon, n.d. Polypad. [Online]
Available at: https://mathigon.org/polypad
[Accessed 15 March 2022].

National Council of Teachers of Mathematics, n.d. Isometric Drawing Tool. [Online]
Available at: https://www.nctm.org/Classroom-Resources/Illuminations/Interactives/Isometric-Drawing-Tool/
[Accessed 15 March 2022].

NSW Government, 2021. Feedback strategies. [Online]
Available at: https://education.nsw.gov.au/teaching-and-learning/professional-learning/teacher-quality-and-accreditation/strong-start-great-teachers/refining-practice/feedback-to-students/feedback-practices-and-strategies#Feedback3
[Accessed 15 March 2022].

Reys, R. E. et al., 2020. Helping Children Learn Mathematics. Milton: John Wiley & Sons Australia..

Siemon, D. et al., 2015. Teaching Mathematics: Foundations to Middle Years. Melbourne: Oxford University Press.

State Government of Victoria, (Department of Education and Training), 2019. Scavenger Hunt. [Online]
Available at: https://fuse.education.vic.gov.au/Resource/LandingPage?ObjectId=0d106473-fe49-45fb-b792-cd7576faaa22
[Accessed 15 March 2022].

State Government of Victoria, (Department of Education and Training), 2020. Classroom talk techniques: Think, pair, share. [Online]
Available at: https://www.education.vic.gov.au/school/teachers/teachingresources/discipline/english/literacy/speakinglistening/Pages/exampleclasstalk.aspx#:~:text=Think%2C%20pair%2C%20share,to%20'turn%20and%20talk'.&text=During%20an%20inquiry%2C%20students%20may,studen
[Accessed 15 March 2022].

State Government of Victoria, (Department of Education and Training), n.d. Describe translations, reflection and rotations of 2D shapes. [Online]
Available at: https://fuse.education.vic.gov.au/MCC/CurriculumItem?code=VCMMG200
[Accessed 15 March 2022].

State Government of Victoria, (Department of Education and Training), n.d. Four Cube Houses. [Online]
Available at: https://fuse.education.vic.gov.au/MCC/CurriculumItem?code=VCMMG198
[Accessed 15 March 2022].

State Government of Victoria, (Department of Education and Training), n.d. Mini Melbourne. [Online]
Available at: https://fuse.education.vic.gov.au/Pages/minimelbourne
[Accessed 15 March 2022].

Sullivan, P., 2017. Challenging Mathematical Tasks. South Melbourne: Oxford University Press.

The Metropolitan Museum of Art, 2001. Geometric Patterns in Islamic Art. [Online]
Available at: https://www.metmuseum.org/toah/hd/geom/hd_geom.htm
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Brush Loads. [Online]
Available at: https://nrich.maths.org/4911
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Colourful Cube. [Online]
Available at: https://nrich.maths.org/11178
[Accessed 15 March 2022].

Van de Walle, J. A., Karp, K. S. & Bay-Williams, J. M., 2017. Elementary and Middle School Mathematics: Teaching Developmentally. Ninth ed. Harlow`(Essex): Pearson Education Limited.