3. Exterior Designs

• Square dot paper: docx PDF
• Kinder square paper
• Computer, laptop or tablet access

This stage aims to develop students’ spatial reasoning and understanding of line and rotational symmetry. Following Architectural Designs, where students created the buildings that represent their dream house and investigated views from different perspectives, this stage allows students to apply their understanding of symmetry to create the top views of buildings.

Introduce students to line and rotational symmetry by playing the video clip, Symmetry on ClickView (sign into ClickView using your department credentials). Complete activities as demonstrated in the video, such as:

Symmetry hunt

Take students on a ‘Symmetry Hunt’ around the school grounds, taking photos of objects they find interesting. Photographs or images from the internet of architecture, artifacts and other objects can also be used. Share the images on an interactive whiteboard or screen and ask students to identify whether the object in the picture is symmetrical or asymmetric. Ask them to identify any lines of symmetry or rotational symmetry.

Create a mathematics word wall using vocabulary and images to describe various forms of symmetry. Encourage students to use the photographs they have taken as a visual prompt to describe reflective and rotational symmetry as well as to identify lines of symmetry on the word wall.

Identifying lines of symmetry

Provide students with images of different objects and shapes. Demonstrate how to use tools such as a plane mirror to determine the lines of symmetry. Ask students to draw lines of symmetry on the images. Encourage students to investigate if letters, numbers, and company logos are symmetrical or asymmetrical and to draw the lines of symmetry for each of them.

Rotational symmetry

Demonstrate how to find the order of symmetry, using the example of the paper windmill in the video clip. A shape has rotational symmetry when it still looks the same after rotating less than one full turn. The number of times the shape matches itself within a full rotation is known as the order of rotational symmetry. Count order of symmetry in the paper windmill.

Challenge students to investigate rotational symmetry in different shapes and letters of the alphabet. Activities can be found in the Mathematics Curriculum Companion to further investigate mirror symmetry and rotational symmetry. 

1. Creating symmetrical designs

In the video clip in the last section, examples of symmetry in architecture were shown. Recap Architectural Designs, in which students explored different buildings from the top view. Recall that some buildings have a symmetrical shape when viewed from the top, such as The Pentagon. Show students satellite images of a city and discuss the types of symmetry they can see in the buildings.  

Introduce students to the Geoboard App, which they will use to create the top view of a building that is symmetrical in shape. It may be useful to allow students to first draw their designs on dot paper or using a physical Geoboard and to show students some examples:

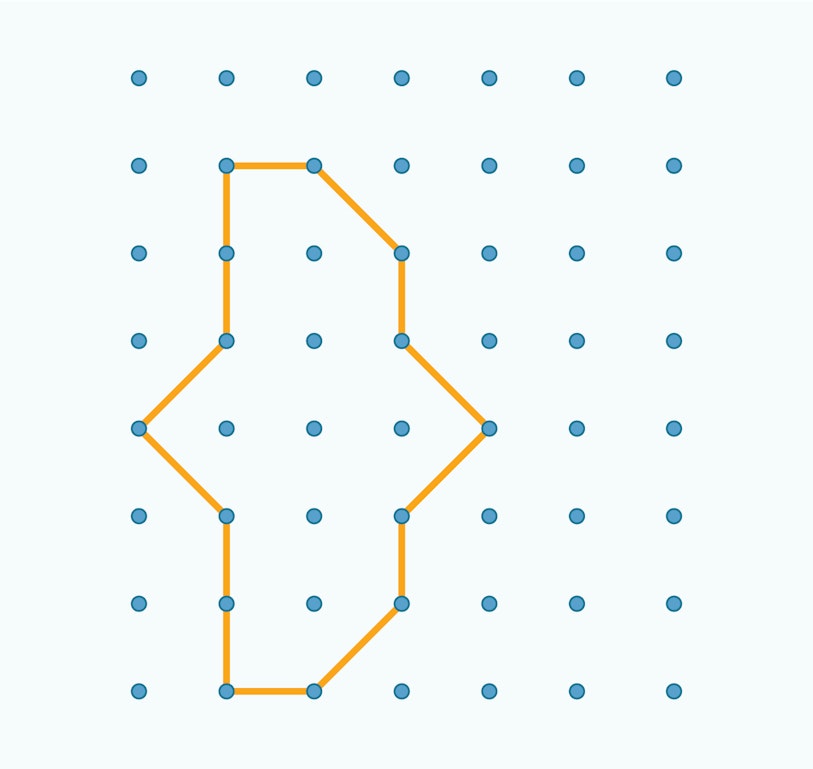
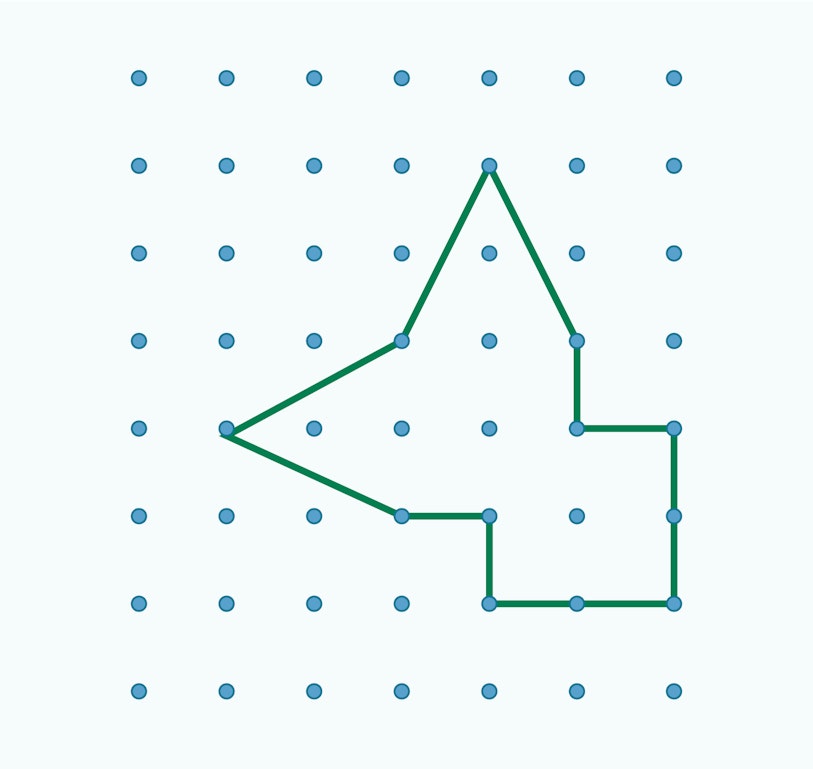
Demonstrate the features of the digital Geoboard, including the drawing tool which allows students to be able to draw a line to join the dots, indicating the line of symmetry. A grid can also be made visible on the geoboard and grid coordinates indicated. Model how to reference coordinate points of each corner of the shape.

Ask students to create various designs with rotational symmetry and different lines of symmetry - vertical, horizontal, and diagonal. Challenge them to create designs with multiple lines of symmetry.

Observe the strategies that students use to solve these problems. Do they first draw lines of symmetry on their paper to guide them?

To check their designs for line symmetry, encourage students to reproduce their design onto a piece of dot paper and have students place a plane mirror on their design so that the mirror is perpendicular to the table. Ask students to look into the mirror to see if the symmetrical mirror image matches their design.

Alternatively, ask students to fold their completed drawing along the line of symmetry and cut out the design on the side with the fold. Ask students to open the paper and observe if either side of the design matches.

Enable students by giving them a plain sheet of paper with a line drawn horizontally or vertically through the middle. Ask students to use eight pattern blocks to make a design on one side of the line, with the blocks touching the line. Once a side is completed, students try to make the mirror image of their design on the other side of the line. Ask students to check for symmetry with a mirror.

To create a design of rotational symmetry, ask students to use one to three pattern blocks to make a design. Guide students to use blocks to show what it will look like if the design is rotated at 90° intervals around a point.

When students are confident with creating symmetrical designs, encourage them to draw on dot grid paper, then use the physical or digital geoboard to create their designs.

Extend students by encouraging them to create designs with rotational symmetry where the angle of rotation is less than 90°. Ask students to measure the angle of rotation. As a further extension, ask students to add grid coordinates to the dot grids and identify the coordinates as the design is rotated around the origin.

2. Creating external designs

Students create a symmetrical building facade using Minecraft: Education Edition. Students also create a symmetrical garden in the front yard, like the one seen in the video clip previously. Encourage students to continue creating symmetrical designs for the exterior of their house, such as the mailbox and streetscape. Look at images of historical homes, such as those at the Chateau de Versailles or Iranian mosques to demonstrate symmetry in building and garden design.

Encourage students to share their designs with their classmates and explain how they are symmetrical. Invite students to identify lines of symmetry and where applicable, the order of rotational symmetry. 

Areas for further exploration

1. Pattern in cultural artefacts

Students to explore various patterns created in artistic and cultural artefacts, such as the motifs in oriental carpets. Websites like Geometric Patterns in Islamic Art can provide a stimulus for discussing symmetry and geometrical transformation in artwork and designs.

2. Logo Licences

Logo Licences is an activity found in the Mathematics Curriculum Companion. Have students investigate symmetry in various logos such as car and company logos. Give students the opportunity to create their own logo that incorporates some form of symmetry.

Creating symmetrical designs

Students create four symmetrical paving designs for areas of their garden. Inform students that the floor tile designs for each area needs to look different from one another, with one having one line of symmetry, another with two lines of symmetry, one with diagonal symmetry and one with rotational symmetry. Provide dotted paper for students to create their paving designs. 

Provide students with tools such as a plane mirror to check their designs for symmetry.

Booker, G., Bond, D., Sparrow, L. & Swan, P., 2014. Teaching Primary Mathematics. Melbourne: Pearson Australia.

BrainPOP, n.d. Blockly: Maze. [Online]
Available at: https://www.brainpop.com/games/blocklymaze/
[Accessed 15 March 2022].

Code.org, 2021. Course E. [Online]
Available at: https://studio.code.org/s/coursee-2021?redirect_warning=true
[Accessed 15 March 2022].

Glenrock Consulting, 2013. How Did They Make Ms. Pac-Man?. [Online]
Available at: https://robertkaplinsky.com/work/ms-pac-man/
[Accessed 15 March 2022].

Google, n.d. Google Maps. [Online]
Available at: https://www.google.com.au/maps
[Accessed 15 March 2022].

Google, n.d. My Maps. [Online]
Available at: https://www.google.com.au/maps/d/u/0/
[Accessed 15 March 2022].

Learning Resources and Technology Services, n.d. Square Dot Paper. [Online]
Available at: https://lrt.ednet.ns.ca/PD/BLM/pdf_files/dot_paper/sq_dot_1cm.pdf
[Accessed 15 March 2022].

Look Cool, 2014. Symmetry. [Online]
Available at: https://online.clickview.com.au/libraries/videos/9550830/symmetry
[Accessed 15 March 2022].

Math Learning Center, n.d. Geoboard. [Online]
Available at: https://apps.mathlearningcenter.org/geoboard/
[Accessed 15 March 2022].

Math Playground, n.d. Area Blocks. [Online]
Available at: https://www.mathplayground.com/area_blocks/index.htm
[Accessed 15 March 2022].

Mathigon, n.d. Polypad. [Online]
Available at: https://mathigon.org/polypad
[Accessed 15 March 2022].

National Council of Teachers of Mathematics, n.d. Isometric Drawing Tool. [Online]
Available at: https://www.nctm.org/Classroom-Resources/Illuminations/Interactives/Isometric-Drawing-Tool/
[Accessed 15 March 2022].

NSW Government, 2021. Feedback strategies. [Online]
Available at: https://education.nsw.gov.au/teaching-and-learning/professional-learning/teacher-quality-and-accreditation/strong-start-great-teachers/refining-practice/feedback-to-students/feedback-practices-and-strategies#Feedback3
[Accessed 15 March 2022].

Reys, R. E. et al., 2020. Helping Children Learn Mathematics. Milton: John Wiley & Sons Australia..

Siemon, D. et al., 2015. Teaching Mathematics: Foundations to Middle Years. Melbourne: Oxford University Press.

State Government of Victoria, (Department of Education and Training), 2019. Scavenger Hunt. [Online]
Available at: https://fuse.education.vic.gov.au/Resource/LandingPage?ObjectId=0d106473-fe49-45fb-b792-cd7576faaa22
[Accessed 15 March 2022].

State Government of Victoria, (Department of Education and Training), 2020. Classroom talk techniques: Think, pair, share. [Online]
Available at: https://www.education.vic.gov.au/school/teachers/teachingresources/discipline/english/literacy/speakinglistening/Pages/exampleclasstalk.aspx#:~:text=Think%2C%20pair%2C%20share,to%20'turn%20and%20talk'.&text=During%20an%20inquiry%2C%20students%20may,studen
[Accessed 15 March 2022].

State Government of Victoria, (Department of Education and Training), n.d. Describe translations, reflection and rotations of 2D shapes. [Online]
Available at: https://fuse.education.vic.gov.au/MCC/CurriculumItem?code=VCMMG200
[Accessed 15 March 2022].

State Government of Victoria, (Department of Education and Training), n.d. Four Cube Houses. [Online]
Available at: https://fuse.education.vic.gov.au/MCC/CurriculumItem?code=VCMMG198
[Accessed 15 March 2022].

State Government of Victoria, (Department of Education and Training), n.d. Mini Melbourne. [Online]
Available at: https://fuse.education.vic.gov.au/Pages/minimelbourne
[Accessed 15 March 2022].

Sullivan, P., 2017. Challenging Mathematical Tasks. South Melbourne: Oxford University Press.

The Metropolitan Museum of Art, 2001. Geometric Patterns in Islamic Art. [Online]
Available at: https://www.metmuseum.org/toah/hd/geom/hd_geom.htm
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Brush Loads. [Online]
Available at: https://nrich.maths.org/4911
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Colourful Cube. [Online]
Available at: https://nrich.maths.org/11178
[Accessed 15 March 2022].

Van de Walle, J. A., Karp, K. S. & Bay-Williams, J. M., 2017. Elementary and Middle School Mathematics: Teaching Developmentally. Ninth ed. Harlow`(Essex): Pearson Education Limited.