4. Duration of Time

• Analogue Clocks
• Paper and pencils
• Empty number lines (created by the students)
• Mathematics Curriculum Companion elapsed time activities
• NRich: Walk and Ride activity
• NRich: How Long Does it Take?
• NRich: Buses 

Ask students, ‘What are some things you might like to do during your school holidays?’ 

Brainstorm some ideas and write these up on the board or on a chart, where students can see the list. Some things on the list might include:

• playing or watching football, soccer or some other sport
• going to the movies
• going for a hike/bushwalk
• doing some cooking
• playing online games
• reading a book
• going camping.

Explain that they will be planning a day where they can choose to do as many of these activities as they would like. Ask students:

How many of these activities could you fit in?

How do you know you could fit them all in?

Students may recognise that it depends upon how long each activity takes, as well as the travel time to and from each event. Explain that the length of time that an activity takes from start to finish is called the duration of the activity.

Encourage students to estimate the duration of certain activities, such as:

• brushing their teeth
• eating lunch
• making their bed
• playing a game of football or other sports
• making a cake
• walking to school.

Ask students the following questions:

• What helped you to estimate the time required for each activity?
• What were you thinking about as you made your estimate?  
• Why is it important to be able to estimate the duration of events?
• Is it important to be able to calculate the exact duration of events?
• If so, is this important for all events, or just some events?
• Why might exact calculation of the duration of events be important?
• How would you go about calculating the exact duration of an event?

Some responses you might expect from students include:

• Subtracting the start time from the finish time
• Skip counting along an analogue clock
• Using a stop watch or timer to measure the elapsed time.

1. Exploring start and finish times

Say to students,

‘If you have decided that one of the activities you would like to do on your holidays is to either attend or watch a sports match, you will need to work out when this match might take place’.

Present the following information to the students:

If a football match takes 2 hours and 15 minutes from start to finish, what might be suitable starting and finishing times? (Sullivan, P & Lilburn, P, 2017, p 74)

Encourage students to work in pairs to promote discussion. Throughout the lesson, ask the following questions:

• How are you going to work out this problem?
• What tools could you use to help you work it out? (E.g., clock, paper & pencil, empty number line)
• Have you found all the possible starting and finishing times? How do you know?

Enable students by simplifying the length of time the game takes (e.g., the football match takes 2 hours to complete).

Extend students by posing the following questions:

• Are all the times that you have come up with practical times to run a football match? Why or why not?
• Describe the advantages and disadvantages of each of the game times that you have chosen.
• What are all the possible starting and finishing times within a 24-hour period?
• Is it possible to list all these times? Why or why not?

2. Calculate the elapsed time

Say to the students,

‘If you have decided to go to the movies as one of your activities during the holidays and you need to get there by bus you do not want to miss the bus, or you may miss your movie. You will need to work out when the bus is scheduled to depart’.

Present the following information to the students:

The time is now 2:45 p.m. The bus leaves at 10 past 4. How long is it until the bus leaves? Work out your answer in two different ways. (Sullivan, P, 2018, p 124).

Students form pairs to work on this problem. Throughout the lesson, ask students the following questions:

• How are you going to work out this problem?
• What tools could you use to help you work it out? (Clock, paper & pencil, empty number line)
• What is the duration of time from now until the bus leaves?

Some possible solutions are outlined (Sullivan, P, 2018, p 125):

• Bridge the hours: 2:45 p.m. to 3:00 p.m. is 15 minutes; it is 1 hour from 3:00 p.m. to 4:00 p.m.; then 4:00 p.m. to 4:10 p.m. is 10 minutes.
• Add the hour: 2:45 p.m. to 3:45 p.m. is 1 hour; then add 15 mins to 4:00 p.m.; then add another 10 minutes more.
• Students may choose to work backwards too. For example: the bus leaves at 10 past 4, go back one hour; it is 10 past 3; then go back 10 minutes; it is 3:00 p.m. Then go back 15 minutes; it is 2:45 p.m. Add the time that you went back to find out how long until the bus comes: 1 hour plus 10 minutes plus 15 minutes equals 1 hour and 25 minutes.

Enable students by explaining that the time is now 2:45 p.m. and that the bus leaves at 5 to 3. Support them work out how long until the bus leaves (Sullivan, P, 2018, p 124).

Extend students by asking them, ‘What’s a rule that would help you with all calculations like this one? (Sullivan, P, 2018, p 124).

3. Exploring duration of time

Say to the students,

‘Gina’s mother is going out, so she has offered to drop Gina to the movies at 2:00 p.m. and she will pick her up again at 4:00 p.m. She does not need to take the bus. Given the duration of time that Gina has at the movie theatre, you need to work out which movie Gina could watch from start to finish before her mother comes to get her.'

The movie schedule is below (NAPLAN, Year 5, 2008).

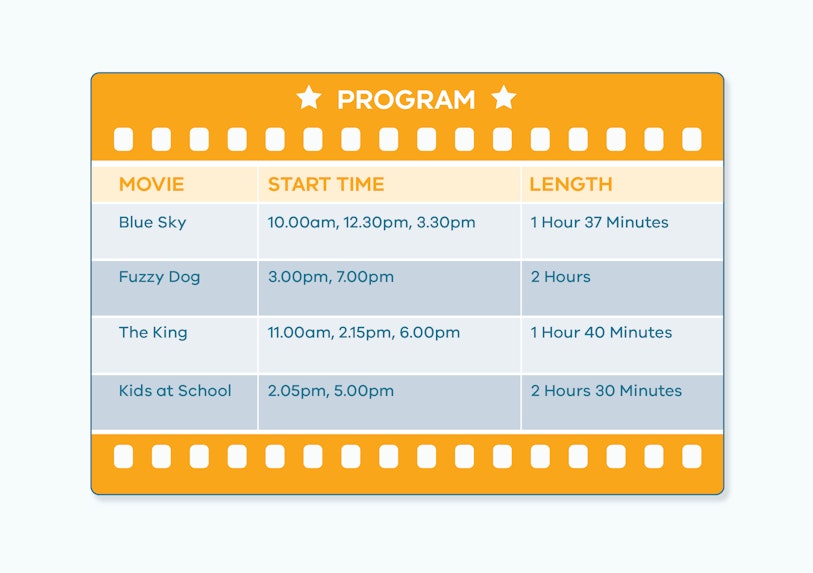
Encourage students to work in pairs to promote discussion. Throughout the lesson, ask the following questions:

• How did you go about working out which movie Gina could watch from start to finish during the duration in which she was at the movie theatre?
• Was there another way that you could work it out?

Enable students by:

• reducing the selection of movies to two, such as Fuzzy Dog and The King
• modifying the starting time to the hour/half hour or simplify the length to the hour or half hour (e.g., 1 ¹/₂ hours long, 2 hours long).

Extend students by asking them to determine three different ways to solve this problem. For example, they may systematically go through each of the times using a number line to jump forwards or backwards, or they may use an analogue clock. They may cross out impossible start times first etc. Ask students to share their strategies with the rest of the class.

4. Further exploration of duration and elapsed time

To expose students to different scenarios regarding calculating duration or elapsed time and using an empty number line, present students with problems that contain the following situations:

• Start and end times are known, duration/elapsed time is unknown
• Start time and duration/elapsed time are known, end time is unknown
• Duration/elapsed time and end time are known, start time is unknown

The Mathematics Curriculum Companion offers additional activities to reinforce student learning of elapsed time. 

In this activity students find elapsed times i.e., the difference from one starting time to another, and learn to record their thinking on empty number lines.

Present the following scenarios to the students:

• Kiarra’s dance concert started at 5:45 p.m. and finished at 10:18 p.m. How long was the concert? (In this question the start and end times are known)
• I started walking the dog at 4:55 p.m., and our walk took 2 hours and 37 minutes. What time did I get home? (Students can count on to determine the finishing time)
• The movie went for 2 hours and 36 minutes. If it finished at 10:05 p.m., what time did it start? (Students can count back on their number line)

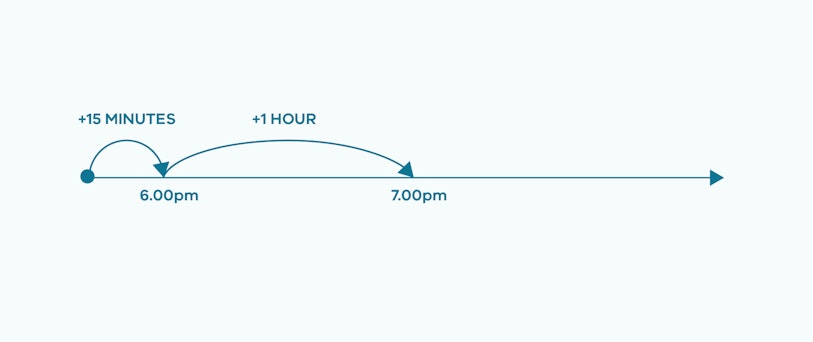
Students should work in pairs or small groups to solve these problems. Throughout the lesson, ask the following questions:

• What strategies did you use to work out each problem?
• Could you use a different strategy to work out these problems?
• Could you work out these problems using another tool, rather than an empty number line?

Some strategies that the students might use with the number line are:

• Jump at half-hour intervals
• Jump to the next hour then add the remaining minutes (this involves using benchmark times, such as knowing that 45 mins is ³/₄ of an hour, therefore a ¹/₄ of an hour is 15 mins).

Enable students by using times that relate to the hour and half hour and using a closed number line to support counting if needed. For example, in the first situation the start time could be 5:30 pm and the end time 10:30 p.m. In the second situation the start time could be 5:00 pm and the walk time could be 2 hours and 30 minutes. In the third situation the movie duration could be 2 hours and 30 minutes and the end time 10:00 p.m. As students increase in confidence, start introducing elapsed times that bridge midday (e.g. the activity starts at 11:00 a.m. and takes an hour and a half). 

Extend students by encouraging them to create their own elapsed time problems, including problems that bridge midday.

Areas for further exploration

• Walk and Ride - NRICH 
• How Long Does it Take? - NRICH 
• Buses - NRICH 

This sequence is focused on building students’ understanding of duration. Duration is the time between the beginning and the end of an event. Duration consists of unbroken units of time that can be large or very small. These units can be used to accurately measure duration/elapsed time.

There are many different scenarios that involve duration and many ways in which to calculate elapsed time, such as counting forwards or backwards using an analogue clock or using a number line. Duration of time may involve the use of mixed units of time, such as minutes and hours. Students need to be able to convert between these different units of time to accurately measure the duration/elapsed time. Understanding that units of time can be thought of as fractions of each other is also useful, for example, 30 minutes is equivalent to ¹/₂ hour.

The activities associated with this stage offer different opportunities for students to engage with understanding and calculating duration/elapsed time. To assess students’ understanding of the concept of duration and how to calculate elapsed time the following can be used:

• Listening and recording notes of students’ responses to questions.
• Reviewing work samples – note the strategies that the students are using to calculate elapsed time.

When reviewing work samples to identify strategies being used by students, check for the following:

• Are they able to use different strategies appropriate to different scenarios involving duration, such as using a clock or number line to count forwards, or count backwards? 
• Are they using benchmarks? 
• Are they adding the hours and then the minutes? 
• Or are they adding to make to the next hour and then adding the remaining minutes? 
• Are they able to convert between different units of time?

Determine whether students understand that estimating elapsed time and calculating the exact elapsed time are both important for different reasons. For example:

• Estimation: If you need to get to school at a specific time, you will need to be able to estimate the duration of the time it will take you to get there, so that you plan appropriately and are on time. Using benchmarks will help with estimating duration. For example, it takes me approximately 15-20 mins to walk one kilometre. My school is 2 kilometres away from home, so it will probably take me 30-40 minutes to walk there. School starts at 9:00 a.m. I should leave home at about 8:20 a.m.
• Exact Calculation: You are making a cake and it needs to bake for 30 minutes. If you put the cake in the oven at 2:15 p.m., you must take it out at exactly 2:45 p.m. or it will burn.

Ask students to reflect on their progress towards achieving the success criteria:

• I can work out the length of an event by comparing the start and the end time of the event
• I can work out the length of an event in a variety of ways, such as skip counting using an analogue clock or jumping forwards or backwards along an empty number line
• I can work out the duration of an event by converting units of time when needed.

As an exit ticket students can write their own duration or elapsed time activity and solve the problem using as many of the above strategies as they can.

Australian Curriculum, Assessment and Reporting Authority, 2005. Numeracy, Year 5. National Assessment Program Literacy and Numeracy (NAPLAN), p. 11.

State Government of Victoria (Department of Education and Training), n.d. Maths Curriculum Companion: Interpret and use timetables. [Online]
Available at: https://fuse.education.vic.gov.au/MCC/CurriculumItem?code=VCMMG226
[Accessed 15 March 2022].

State Government of Victoria (Department of Education and Training), n.d. Maths Curriculum Companion: Measure, calculate and compare elapsed time. [Online]
Available at: https://fuse.education.vic.gov.au/MCC/CurriculumItem?code=VCMMG227#Resources
[Accessed 15 March 2022].

Sullivan, P., 2017. Challenging Mathematical Tasks. South Melbourne: Oxford University Press.

Sullivan, P. & Lilburn, P., 2017. Open-Ended Maths Activities: Using ‘Good’ Questions to Enhance Learning in Mathematics. revised ed. Australia: Oxford University Press.

Thomas, M., 2018. A matter of time: an investigation into the learning and teaching of time in the middle primary years. Australian Catholic University: Unpublished Doctoral Thesis.

Thomas, M., 2020. A Matter of Time. Prime Number, 35(1).

Thomas, M., Clarke, D., McDonough, A. & Clarkson, P., 2016. Time: Assessing Understanding of Core Ideas: Opening up mathematics education research (Proceedings of the 39th annual conference of the Mathematics Education Research Group of Australasia). Adelaide, MERGA, pp. 592-599.

Thomas, M., Clarke, D., McDonough, A. & Clarkson, P., 2016. Understanding Time: A Research Based Framework: Opening up mathematics education research (Proceedings of the 39th annual conference of the Mathematics Education Research Group of Australasia. Adelaide, MERGA.

University of Cambridge, n.d. NRICH: Buses. [Online]
Available at: https://nrich.maths.org/2305
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: How Long Does it Take?. [Online]
Available at: https://nrich.maths.org/10343
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Walk and Ride. [Online]
Available at: https://nrich.maths.org/985
[Accessed 15 March 2022].