2. Measurement of Time

• Clocks (analogue and digital)
• Calendars
• Timers
• Strips of paper, pens/pencils
• Thinkboard template: docx PDF
• Brainstorm template: docx PDF
• What time is it activity sheet: docx PDF
• Four main moon phases activity sheet: docx PDF
• An interactive online Math Clock from The Math Learning Center
• Resources for teaching time from the Mathematics Curriculum Companion
• Two Clocks NRICH activity from the University of Cambridge
• Wonky Watches NRICH activity from the University of Cambridge

Review the learning from previous lessons about how years, months, weeks, and days were determined, and how a calendar is used to measure these different units of time. Discuss how a day, which is the smallest unit measurable using a calendar, can be divided into smaller time units. Ask students what tools we use to measure the time units that are smaller than a day. Record responses on an anchor chart. Explain to students that we commonly use clocks to measure smaller units of time - hours, minutes, and seconds. 

Provide each student with a blank piece of paper. Ask students to draw an analogue clock, and to choose a time to show on the clock, writing that time in words at the top of the page. Students then draw a digital clock underneath, showing the same time. Use the drawings to identify student's entering knowledge about time and clocks, and to determine specific learning needs. For example, do students space the numbers 1-12 correctly around the analogue clock? Do they show the time accurately with drawn on hands? Can the represent their chosen time on both the analogue and digital clock correctly? Can they name the time correctly?

Engage students in a discussion about clocks and record responses on an anchor chart. Prompts may include:

• Analogue clock and parts (numbers, minute marks, hour hand, minute hand, second hand)
• Digital clocks
• a.m. and p.m.
• 24-hour time
• Using clocks to read time (different ways to read time, including fractional time, e. g. 3:15, ¹/₄ past 3, 1515, 6:45, ¹/₄ to 7, 1845)
• Using clocks to calculate duration of time.

Present students with an image of an analogue clock with no numbers.

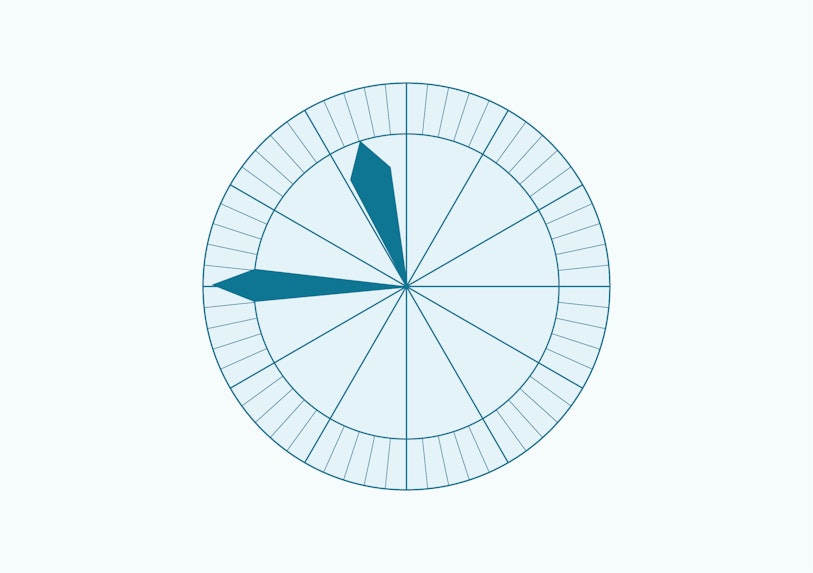
Start a group discussion by asking students:

'Imagine that this clock was dropped on the floor, and we do not know which way around it should go. What time might it be showing? How do you know?’

Provide time for students to observe the clock, then ask them to share their responses and reasoning. Record on the board or an anchor chart.

Ask students the following questions:

• What parts of an analogue clock are essential for someone to read the time accurately?
• Do you need numbers?
• Do you need all hands to be present? If not, which one(s) do need to be present?
• What are some different ways that you can read the time? (8:15, ¹/₄ past 8)
• What are some other questions we could ask about this clock or about time in general?

Enable students by pointing to the line on the clock that represents 12. Ask the students to tell you what the time the clock is showing now that they have this information.

Extend students by asking them, ‘What are all the possible times this clock could be showing? Have you found them all? How do you know?’

Discuss features of analogue clocks with students (e.g. they are often round but sometimes may be other shapes). The traditional analogue clock circle can be divided up into fractions, and that time is often represented or labelled according to the fractional amount that the minute hand has moved around the clock (circle). Tell students that the next activity will explore time in fractions.

1. Fractions of time

In this activity from the Mathematics Curriculum Companion, students learn about fractional parts of time, working with halves, quarters, twelfths, thirds, fifths, and tenths.

Explain to students that they will find fractional parts of different times and express them in terms of minutes and hours. Show students a rectangle strip divided into 60 equal segments to represent a full hour with 60 minutes. Each division shows one minute. Have a clock available so that students can link the clock to the example shown below, using rectangular strips.

Demonstrate to students how to determine:

• What is ⁴/₅ of one hour?

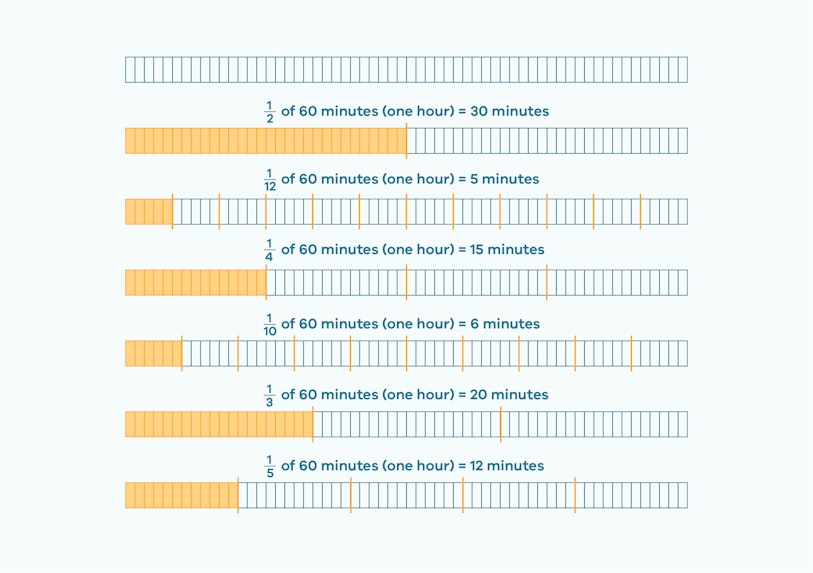
Divide the strip, marking fifths of an hour (12 minutes). Think aloud to model how you determined this (for example, 'I know ¹/₁₀ of 60 minutes is 6 minutes, therefore ¹/₅ of 60 minutes must be 12 minutes').

Complete the following worked example:

It takes me ³/₁₂ of an hour to walk to school. If I leave home at 8.30 am what time will I arrive at school? ³/₁₂ is the same as ¹/₄ of an hour. One hour is 60 minutes. One quarter of 60 minutes is 15 minutes. Therefore, it takes me 15 minutes to walk to school. If I leave at 8:30 a.m., I will arrive at 8:45 am (each step can be demonstrated using an analogue clock or a rectangular strip).

Provide duration questions for students to respond to using their time strips. For example:

• If I left Melbourne Central train station at 12:15 p.m. and it took ⁴/₁₂ of an hour to get to Altona station, what time would I arrive at my destination? How long was the trip?
• My active training app is set for me to complete one hour of exercise each day. If I completed a daily activity of 120% (note that 120% is the same as ¹²/₁₀) how much exercise did I do in one day? If I started exercising at 11:48 a.m., what time did I finish?'

2. Understanding the structure of clocks and different ways to read time

Use the Two Clocks activity from the University of Cambridge.

Arrange students into pairs or small groups and present them with this task, which consists of a series of clocks. The first series has the minute hand missing, and the second hand has the hour hand missing. Tell the students that you would like them to work out the time on these clocks, even though one hand is missing. Start with the first set of clocks where only the hour hand is present on the clock.

Ask students the following questions:

• Do you think you can work out the time even though one hand is missing?
• What do you need to look for, to help you work out the time?
• What does this hand tell you?
• What time do you think each clock is showing? How do you know?

In pairs or groups, students answer the questions above, as well as the questions outlined in the activity. Students justify their answers to each of these questions. Ask the students to add the missing minute hand to each of the clocks, and then to record the digital time under each of the clocks. Ask them to record these times in as many ways as they can (for example, 10 minutes to 12 or 11:50; 6:30 or half past 6).

Before beginning the second part of this activity, gather the students together and ask a couple of groups to present their answers along with justification for their answers.

In the second part of the activity, give the students a set of the clocks with only the minute hand showing and a copy of each of the scenarios. Tell students that they need to cut out and match the scenarios to the correct clock face. Ask the students to add the missing hour hand to each of the clocks, and to record the digital time under each of the clocks. Students record the time in as many ways as they can (for example, 10 minutes to 12 or 11:50; 6:30 or half past 6)

Ask students the following questions:

• What does that hand tell you?
• What time could the clock be showing? How do you know?
• Roughly, what could the time be on Monday/Tuesday etc?
• Which three clocks show minute hands five minutes apart?
• How might that help you to decide which days they were?

At the end of this session, gather the students together again, and ask a couple of different groups to share their responses and thinking.

Enable students by asking them to complete only the first part of the activity which includes the hour hand. Demonstrate to students how to mark on the time of 3:30 p.m. on the clock face, indicating the time when school finishes on the second set of clocks to act as a reference point.

Extend students by asking them to create two more questions for the first series of clocks to swap with another group. Students draw the clocks with the hour hand in the correct corresponding position. Ask students to convert the digital times they recorded into 24-hour times. Explain that in many countries they use 24-hour time rather than 12-hour time. Ask students to share other times where 24-hour time is used. 

3. The hand fell off my clock

This is a consolidation task to help students to understand the structure of clocks and different ways to read time.

Show students the clocks above and say:

‘When I woke up this morning, I noticed that one hand had fallen off my clock. I went back to sleep and when I woke up the second time, the clock hand had moved.’

Ask the students:

• Which picture shows the first clock I saw?
• Which hand has fallen off my clock?
• What time might it have been?
• What time might the other hand show?
• Is there only one solution?

4. Using clocks to calculate duration (elapsed time)

This activity is based on the Wonky Watches activity from the University of Cambridge.

Arrange students into pairs or small groups and introduce the task:

Mandeep's watch loses two minutes every hour.

Adam's watch gains one minute every hour.

They both set their watches from the radio at 6:00 a.m. then start their journeys to the airport. When they arrive (at the same time) their watches are 10 minutes apart.

At what time (the real time) did they arrive at the airport?

During the activity, prompt students with the following questions:

• How will you work this out?
• What strategy will you use?
• Is there another way that you would work this out?
• How do you know that your answer is correct? Can you prove it to me?

5. Using problem-solving to calculate the correct time

Arrange the students into pairs or small groups and present them with this task (created by Doug Clarke & Laurinda Lomas).

Explain to students that there are three clocks, and each clock shows a different time:

• one clock is 3 hours 10 minutes fast
• one is 5 hours fast
• one is 2 hours 5 minutes slow.

Students determine the correct time using the clues provided. Explain that they need to record how they worked out the problem. Encourage them to use the following interactive digital tool to make their recordings: Math Clock by The Math Learning Center. 

During the activity, prompt students with the following questions:

• How will you work this out?
• What strategy will you use?
• Is there another way that you would work this out?
• How do you know that your answer is correct? Can you prove it to me?

Monitor students' understanding by:

• making notes on whether they are using the problem-solving process - understand the problem, plan their strategy, carry out the plan, and then check it over
• roaming around and observing the different groups. Make notes regarding the strategies that they are using. If they are having difficulty, you might suggest a specific strategy they could try, such as working with the hours first, and then adjusting their answers after adding in the minutes.
• collecting and looking at the students’ recordings on the above link (Math Clock by The Math Learning Center)

There is one correct time, but there are several ways in which the correct time can be determined.  When students have completed the activity, select pairs/groups who have used different strategies to share how they worked out the problem. For example, some students may choose a trial-and-error method. Others may use a systematic approach incorporating a chart, while others may break it down and work with the minutes or the hours first to try to find the correct time. Some may use the clocks themselves to count forwards or backwards, or some may choose to use a number line. Record the different ways in which the same problem can be solved on an anchor chart.

6. Relationship between units of time (converting between units)

Students work in pairs or small groups, analysing the following True or False statements (Sullivan, 2018, p. 127):

• There are 720 hours in January.
• There are 178 hours in 1 week.
• There are 1440 minutes in 1 day.
• There are 360 seconds in 1 hour.
• There are 44,640 seconds in March.

Ask students to work out whether these statements are true or false, and to explain their reasoning. Have clocks, calendars, and calculators available for students to use if needed. The Math Clock interactive digital tool by The Math Learning Center may also be useful to students. At the end of this session gather the students together and ask a couple of groups to present their answers along with justification for their answers. 

Ask students:

• What time units/instrument did you need to use to measure time?
• What did you need to know about units of time to answer these questions, or determine whether a statement was true or false?

Additional problems

Highlight to students the need to explain their thinking and how they worked out each problem.

• How many days from now until January 1?
• How many sleeps from now until your birthday?
• What day is it 150 hours from now?
• What is the time 30 hours from now?
• What was the date 40 days ago?
• What will be the time in 3600 seconds?

Ask students:

• What time units/instrument did you need to use to measure time?
• What did you need to know about units of time to answer these questions, or determine whether a statement was true or false?

Areas for further exploration

Exploring 24-hour time:

• Five on the Clock

Exploring centuries and millenniums:

• Taking Time - New Zealand Maths 
• Thousands and Millions - NRICH (Fermi Questions)  
• The New Millennium - NRICH 

This sequence is focused on furthering students’ understanding of different time measuring devices, how they work and how they are related. At the completion of the sequence, students should be able to explain that a calendar is used when measuring bigger units of time, such as years, months, weeks, and days and that these time units are related to the revolution of the Earth around the Sun and the Moon around the Earth.

Students should be able to explain that when using units of time that are smaller than a day, clocks are used to measure these units, which consist of hours, minutes, and seconds and are related to the rotation of the Earth on its axis. Students should recognise different types of clocks, such as analogue and digital clocks, and can represent times in a variety of ways, such as 12-hour time (using am and pm), 24-hour time, and representing time fractionally. As students understand the relationship between different units of time, they use this knowledge to convert between units so that they can accurately calculate duration/elapsed time. 

Clocks and calendars follow a pattern of equivalent units of time that are repeated. Each cycle represents the duration of that time unit. For example: the minute hand of a clock goes around the clock face every hour, at the end of the week, the days repeat themselves. Duration of events are measured and represented by these units and may encompass partial units or successive units. Each calendar year is labelled chronologically and does not repeat. This represents the succession of time.
 

The activities associated with this stage offer different opportunities for students to engage with understanding time in addition to measuring devices and how to use these. To assess students’ understanding of these and the relationship between the different units of time represented by these different devices the following can be used:

• Listening and recording notes of students’ responses to questions.
• Listening and making notes about the language they are using, such as a.m., p.m., 24-hour times, quarter to, half past etc.
• Looking at work samples – note the reasoning that students use to justify their answers.

At the end of this session ask the students to complete a Thinkboard or Brainstorm. Templates are available in the Materials and texts section. This can be used as part of your assessment on students’ understanding of time measurement. In the centre of each template, students should write the words 'clocks and calendars’.

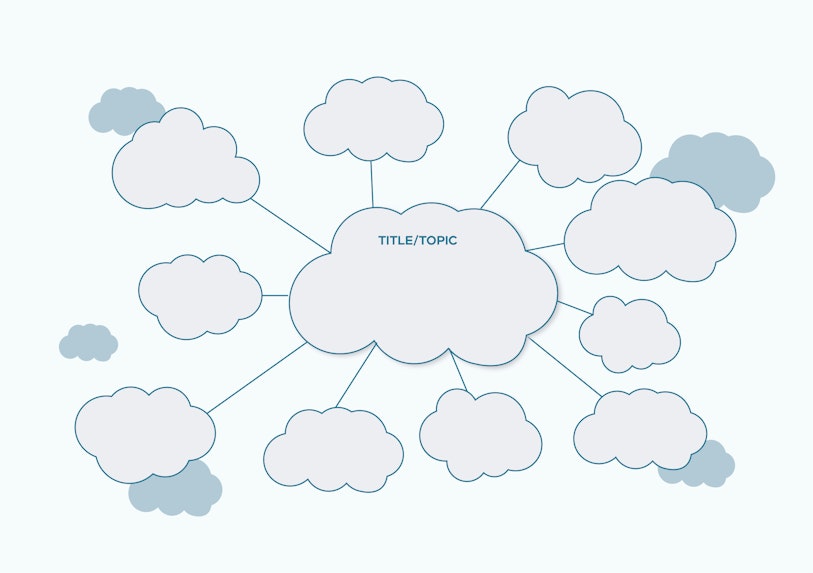
The Brainstorm template offers students an open opportunity to record what they know about each of these time measuring devices.

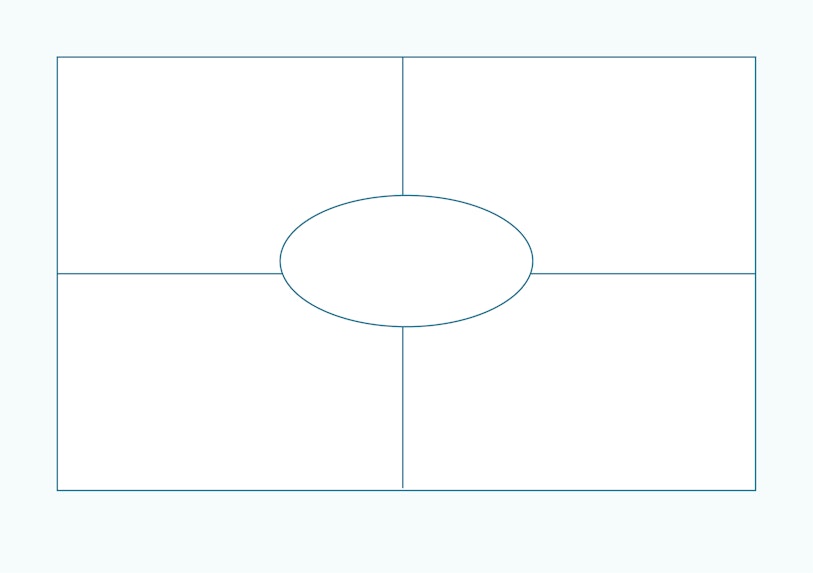
If using the Thinkboard template, include headings for each section to guide students' responses, such as 'examples of clocks and calendars', 'units of time', 'conversion of time', and 'examples of fractional times'. 

Downton, A., Knight, R., Clarke, D. & Lewis, G., 2015. Mathematics Assessment for Learning: Rich Tasks & Work Samples. 3 ed. Melbourne: Australian Catholic University.

Math Learning Center, n.d. Math Clock. [Online]
Available at: https://apps.mathlearningcenter.org/math-clock/
[Accessed 15 March 2022].

New Zealand Government, n.d. nzmaths: The home of mathematics education in New Zealand. [Online]
Available at: https://nzmaths.co.nz/
[Accessed 15 March 2022].

New Zealand Government, n.d. Taking Time. [Online]
Available at: https://nzmaths.co.nz/resource/taking-time
[Accessed 15 March 2022].

State Government of Victoria (Department of Education and Training), n.d. Maths Curriculum Companion: Measure, calculate and compare elapsed time. [Online]
Available at: https://fuse.education.vic.gov.au/MCC/CurriculumItem?code=VCMMG227
[Accessed 15 March 2022].

Thomas, M., 2018. A matter of time: an investigation into the learning and teaching of time in the middle primary years. Australian Catholic University: Unpublished Doctoral Thesis.

Thomas, M., 2020. A Matter of Time. Prime Number, 35(1).

Thomas, M., Clarke, D., McDonough, A. & Clarkson, P., 2016. Time: Assessing Understanding of Core Ideas: Opening up mathematics education research (Proceedings of the 39th annual conference of the Mathematics Education Research Group of Australasia). Adelaide, MERGA, pp. 592-599.

Thomas, M., Clarke, D., McDonough, A. & Clarkson, P., 2016. Understanding Time: A Research Based Framework: Opening up mathematics education research (Proceedings of the 39th annual conference of the Mathematics Education Research Group of Australasia. Adelaide, MERGA.

University of Cambridge, n.d. NRICH: 5 on the clock. [Online]
Available at: https://nrich.maths.org/1981
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Mathematics Resources for Teachers, Parents and Children. [Online]
Available at: https://nrich.maths.org
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: The new Millennium – when did it begin?. [Online]
Available at: https://nrich.maths.org/2560
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Thousands and Millions. [Online]
Available at: https://nrich.maths.org/6046
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Two Clocks. [Online]
Available at: https://nrich.maths.org/4806
[Accessed 15 March 2022].

University of Cambridge, n.d. NRICH: Wonky Watches. [Online]
Available at: https://nrich.maths.org/1002
[Accessed 15 March 2022].

Van de Walle, J., Karp, K., M, B.-W. J. & Brass, A., 2019. Primary and Middle Years Mathematics: Teaching Developmentally. Australia: Pearson.