4. Circles: Establishing Area Formula

• Discussion chart: jpeg
• Discussion resource: jpeg
• Circle on grid paper: jpeg
• Demonstration resource: jpeg

This stage of the sequence builds on the previous stage, Establishing Pi, by giving students the opportunity to apply their knowledge of the relationship between the circumference and the diameter of a circle to analyse why the area formula of a circle is:

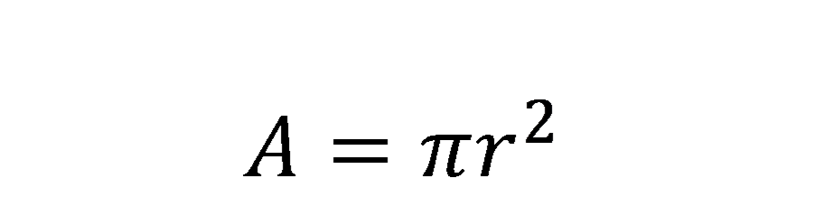
These activities are adapted from the Area of a Circle sequence from Resolve teaching resources.

Introduce students to the concept of ‘radius square’ by first asking them to draw a circle and draw a radius. Ask students to draw a square using the radius as the square’s edge, for example:

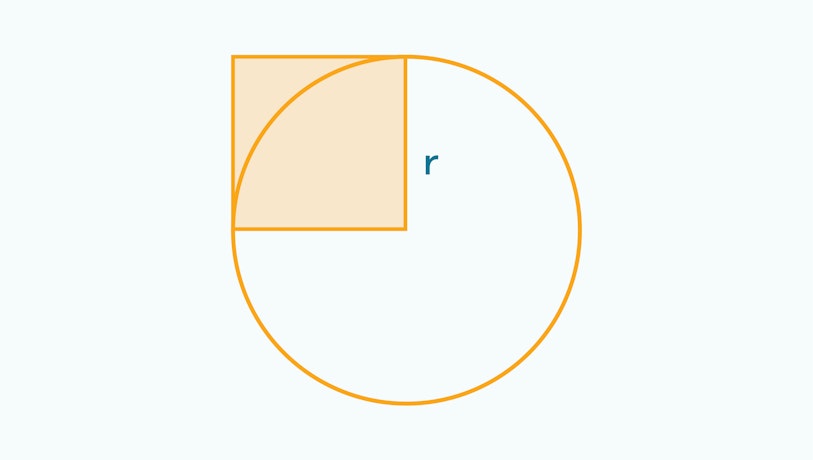
Pose the following questions:

• What is the area of the radius square?
• How many radius squares will fit inside the circle?

Enable students by suggesting that they make two identical drawings of their radius square and cut out one set. Hint to students that they can cut their radius square in half to figure out how many of the pieces can fit into the circle.

Support students to determine that four halves of a radius square will fit inside the circle, which equates to two radius squares.

Students work collaboratively with their peers in pairs or small groups on the following problems:

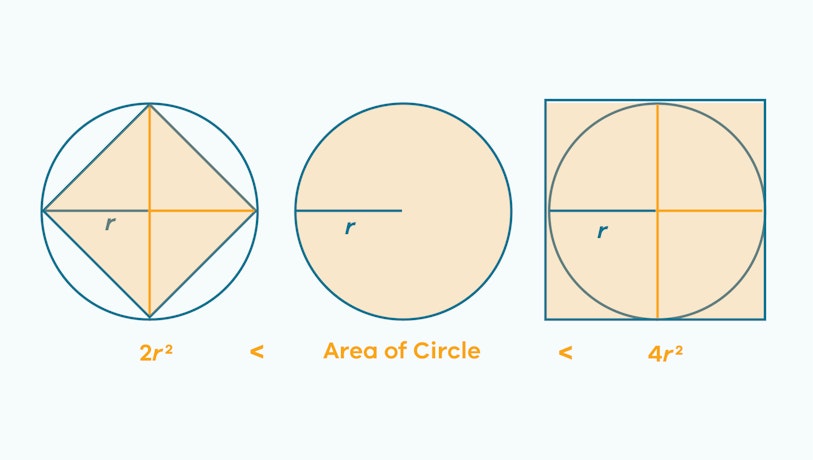
• Draw a diagram to explain why the area of the circle is smaller than the area of four radius squares.
• Draw a diagram to explain why the area of the circle is greater than the area of two radius squares.

Ask students to form a generalisation about the area of the circle compared to its radius square. When students realise that the area of the circle is bigger than two squares of length r, and smaller than four squares of length r, they will probably guess that the exact factor is pi.

Ask students to work collaboratively in small groups on one or more of the following activities to explore areas of circles that build conceptual understanding for the formulas.

As students are working in their groups, encourage them to discuss with their peers how these activities help them explain why the area formula for circles is:

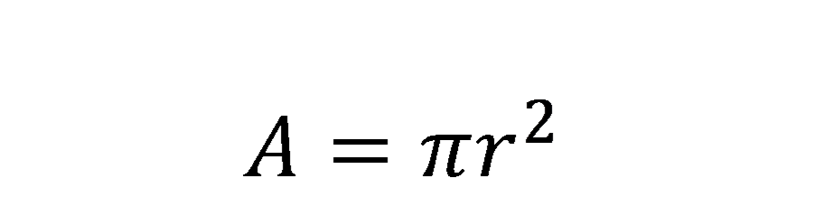
Encourage students within each group to prepare a presentation to explain their understanding to the rest of the class.

1. The corner square

This activity refines the estimation shown in the 'Get started' phase, that the area of a circle is somewhere between 2 and 4 radius squares. Students count squares to find the ratio of the area of the circle to the area of the ‘corner square’. 

Provide students with a copy of a circle drawn on grid paper (see Materials and texts section above), with a radius square marked out, and ask them to discuss:

• If the radius of the circle is r, what is the area of the shaded ‘corner square’?
• How can you use the grid to work out how many ‘corner squares’ fit into the circle?

Students may reach an estimate that the number of radius squares needed is about 3.1 or 3.2. Note that this activity does not prove the area formula of a circle, but does give an approximation.

Enable students by giving them another copy of the template and suggesting that they could cut out the square units of the ‘corner square’.

Extend students by asking, “Can you use another way to work out how many ‘corner squares’ fit into the circle?"

2. Slices of Pie

This activity allows students to visualise the area of circles by cutting a circle into sectors and rearranging them to look more like a parallelogram.

Have students divide a circle into equal sectors or present them with a copy of the discussion chart (see Materials and texts section above) showing a circle cut into 18 sectors. Ask students to cut out the sectors and rearrange them so they resemble a parallelogram, or almost like a rectangle.

Discuss the following:

• What will happen to the shape of the ‘almost rectangle’ when you increase the number of sectors of the circle?
• Why is the length, or base, of the ‘almost rectangle’ pi x r and the width, or height, r?
• How could you use this model to describe the area formula of a circle?

A common misconception is that when students think of the area of the circle as:

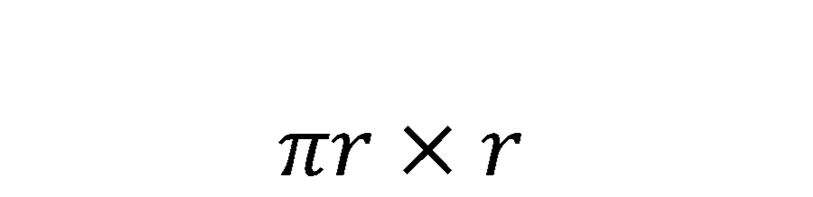
they write the formula as:

Guide students to distinguish between the meaning of "twice a number" and "the square of a number". For example:

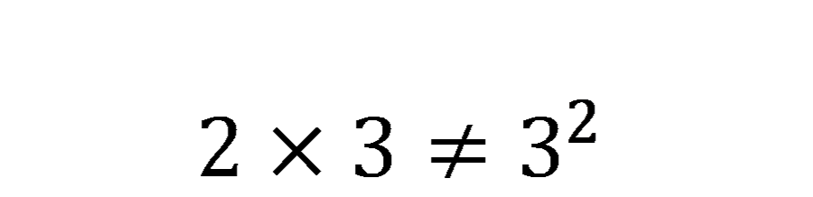
Enable students by prompting them to use the information they know about the formula for circumference of a circle to determine the length of the ‘almost rectangle’.

After students have completed the activity, It may be useful to present students with a demonstration video of segmenting a circle and rearranging the segments into an ‘almost rectangle’.

3. Archimedes’ polygon

This activity demonstrates the Greek mathematician, Archimedes’ proposition, around 250 BCE, that the area of the circle is equal to the area of a right-angled triangle, whose height is equal to the radius of the circle and whose base is equal to its circumference.

Present students with a copy of the discussion resource, with the following diagrams shown:

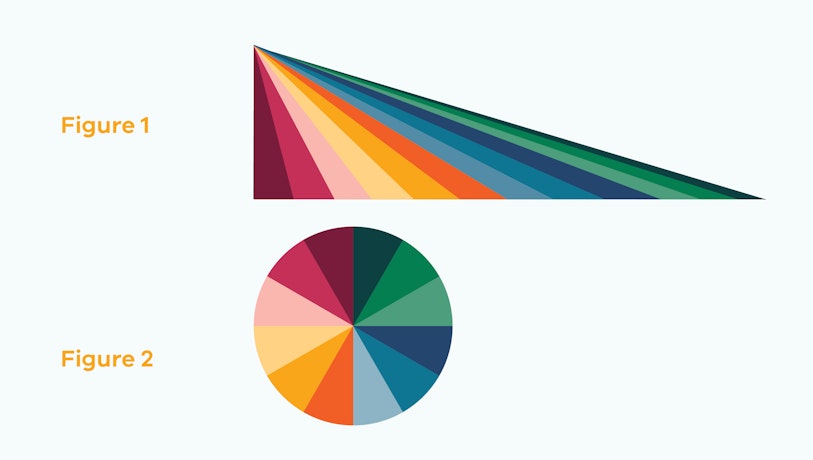
Figure 1 shows a right-angled triangle. One of the sides has the length r (radius of the circle) and the other side has the length C (circumference of the circle). The side of length C is divided equally into a given number of line segments. In the example diagram, it is divided into 12 segments.

Figure 2 shows a circle of radius, r, and divided into the same number of sectors as line segments constructed in Figure 1, with the vertex of each sector at the centre of the circle.

Have students discuss the following in groups:

• Why is the area of each small triangle in the large right-angled triangle the same?
• Students should recognise that triangles of the same height and equal bases have the same area. What happens to each sector of the circle when you increase the number of line segments along the base of the large triangle?

Students should recap from previous lessons and observe that as the number of sectors and line segments increases, the area of each sector becomes nearly the same as the area of the triangle constructed on each line segment.

• How can this demonstration prove that the formula for the area of the circle is:

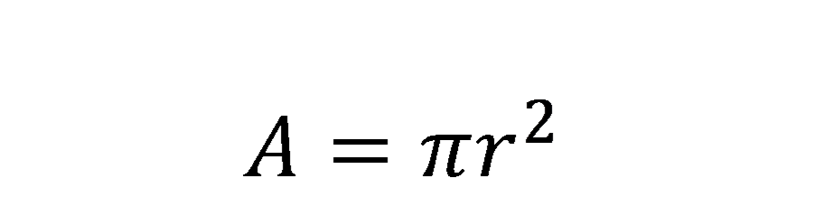
Enable students by supporting them to identify the length of the base of each triangle and to recall the area of triangles.

4. Unravelling the circle

Provide a copy of the demonstration resource (see Materials and texts section above) to the students and explain that in the diagram shown, the circle is visualised as being composed of a series of concentric rings. It is cut along a radius and the rings become near rectangles that can be laid one beside the other to form an ‘almost triangle’.

Have students in the groups discuss the following:

• What happens when you decrease the width of the rings in the circle?
• What will be the length of the base of the ‘almost’ triangle and what is its height?
• How can you use this model to explain the area formula for a circle?

 When students have gained understanding of how the area formula of circles are derived, encourage them to find the area of various circles or circular objects such as those used in Activity (i) – Plates, Balls and Other Round Things of the Establishing Pi stage.

Areas for further exploration

1. Bullseye

The Bullseye activity from Nrich Maths focuses on applying students’ understanding of the area of circles to solve problems.

Students are presented with an illustration of target, such as: 

When the radii of each circle are known, students can determine the fraction and percentage of the circle that each colour is in.

Students’ presentations explaining their understanding of how the area formulas for circles are derived could be used as formative assessment of student learning. Consider the following:

• How did the students connect the visual model to the algebraic formula for area of a circle?
• Were the students able to explain the mathematical basis for deriving the area of a circle?
• Were they able to explain why the formula for area of a circle works to solve for area of all circles?

To further assess students’ understanding of the relationship between the circumference, radius, diameter and area, pose the following questions:

• Given the circumference of a circular object, how can you identify the area of this object? Justify your answers with mathematical thinking and using manipulatives.
• Given the area of a circular object, how can you identify the circumference of this object? Justify your answers with mathematical thinking and using manipulatives.

Australian Academy of Science, 2020. Area of a Circle. [Online]
Available at: https://www.resolve.edu.au/area-circle
[Accessed 15 March 2022].

Mathematicsonline, 2011. Area of a circle, how to get the formula. [Online]
Available at: https://www.youtube.com/watch?app=desktop&v=YokKp3pwVFc
[Accessed 15 March 2022].

Reys, R. E. et al., 2020. Helping Children Learn Mathematics. Milton: John Wiley & Sons Australia..

Siemon, D. et al., 2015. Teaching Mathematics: Foundations to Middle Years. Melbourne: Oxford University Press.

University of Cambridge, n.d. NRICH: Bull's Eye. [Online]
Available at: https://nrich.maths.org/780
[Accessed 15 March 2022].

Van de Walle, J., Karp, K., M, B.-W. J. & Brass, A., 2019. Primary and Middle Years Mathematics: Teaching Developmentally. Australia: Pearson.