1. Quadrilaterals: Investigating Areas of Trapeziums

• 1 cm grid paper: PDF

The following activity is adapted from the ‘Making Connections – Similarities and Differences’ lesson published by the Government of South Australia (2017).

Show students a small selection of shapes on grid paper, such as:

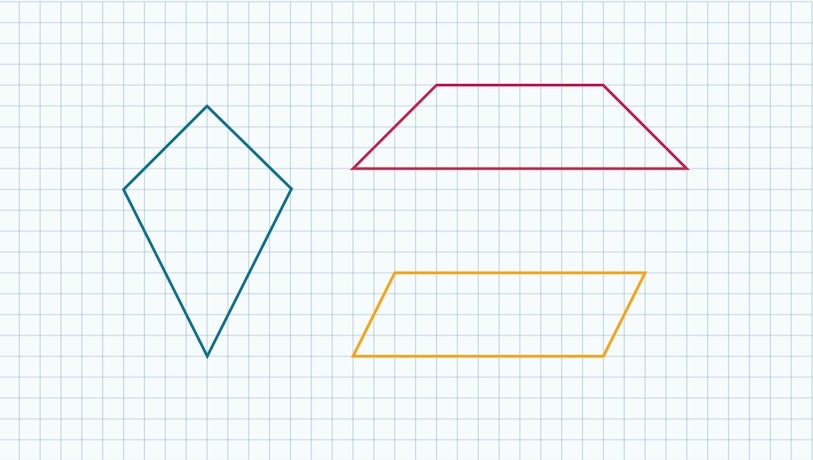
Generate discussion about the properties of these shapes by asking students, “Which is the odd one out?” Encourage students to express their opinion, explain their reasoning for their choices and to provide alternate points of views.

Support students to refer to the shapes using the appropriate mathematical terminology, teaching any unfamiliar vocabulary as needed. Prompt students to focus on the properties of a trapezium, kite and parallelogram and notice how they are similar or different.

Student responses may include:

• the parallelogram is the only one with rotational symmetry
• the kite is the only shape with a right angle
• the kite does not have pairs of parallel lines
• the parallelogram is the only one with two pairs of parallel lines.

1. From rectangles to parallelograms

Finding the area formulas of rectangles and parallelograms is included in the Year 7 content descriptions. This activity aims to recap students’ understanding of the similarities between parallelograms and rectangles.

Review the area formula of parallelograms by presenting the following problem:

Using a 1 cm grid paper, how many different parallelograms can you make with an area of 12 square centimetres?

Invite students to work collaboratively with a partner and observe their approach to solving the problem. Do they count squares and part squares? Do they use their prior knowledge of the relationship between rectangles and parallelograms to draw the shapes?

If necessary, prompt students to transform a rectangle of 12 square centimetres into a parallelogram. Students may recall that a parallelogram can be formed by cutting a diagonal line from one corner of the rectangle and rearranging this triangle piece to the other end of the rectangle, as shown below:

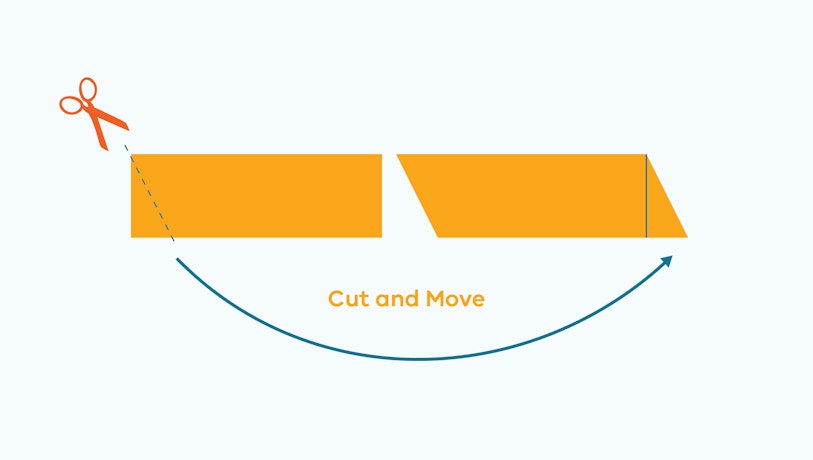
Ask students:

• What can you tell me about the area of the rectangle and the area of the parallelograms?
• Using the words 'base' and 'height', can you write a formula for finding the area of parallelograms?
• How can you label the parallelogram? Which is the base? Which is the height?

Notice if students mistakenly label the slanted side of the parallelogram as its height. If this is so, guide students to identify the height of a rectangle and correspond this to the height of a parallelogram:

Ask students:

"What if the lengths of every side of the parallelogram are equal?"

When the lengths of all sides are equal, a parallelogram is a rhombus. Students will explore another way of finding the area of rhombuses in the next stage of this learning sequence.

Enable students by first discussing what parallelograms are, then asking them to draw a parallelogram with an area of 2 square centimetres on grid paper.

Extend students by asking them to convince you that they have drawn all possible different parallelograms with an area of 12 square centimetres.

2. From parallelograms to trapeziums

Review the properties of trapeziums by asking students to create at least four different trapeziums using a Geoboard, using the Geoboard App, or by drawing the trapeziums on grid paper. Examples of trapeziums include:

Pose the following problem:

Find the area of each of the trapezium using 2 different methods.

Students work collaboratively with their peers in pairs or in small groups.

Students may find the area of a trapezium in various ways, each related to decomposing the trapezium into a simpler shape or combination of shapes where ways to find these areas are already known. Then the area of the trapezium is the sum of the areas of these shapes. For example:

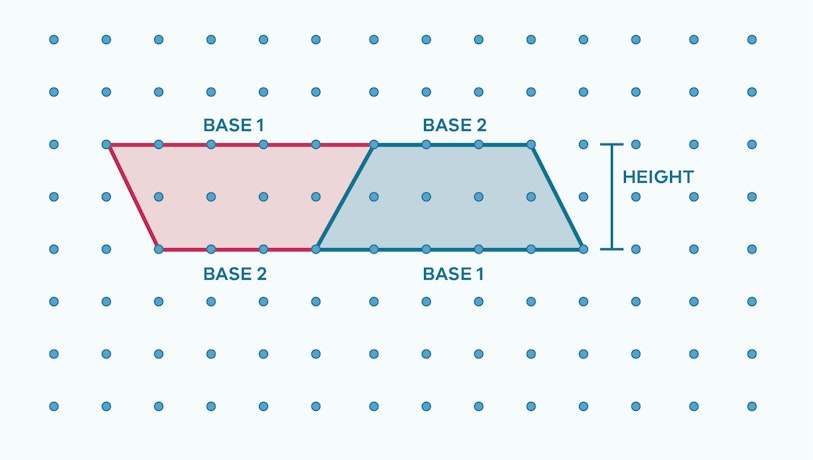
• Combining two congruent trapeziums to form a parallelogram with the same height and a base equal to the sum of the bases in the trapezium.

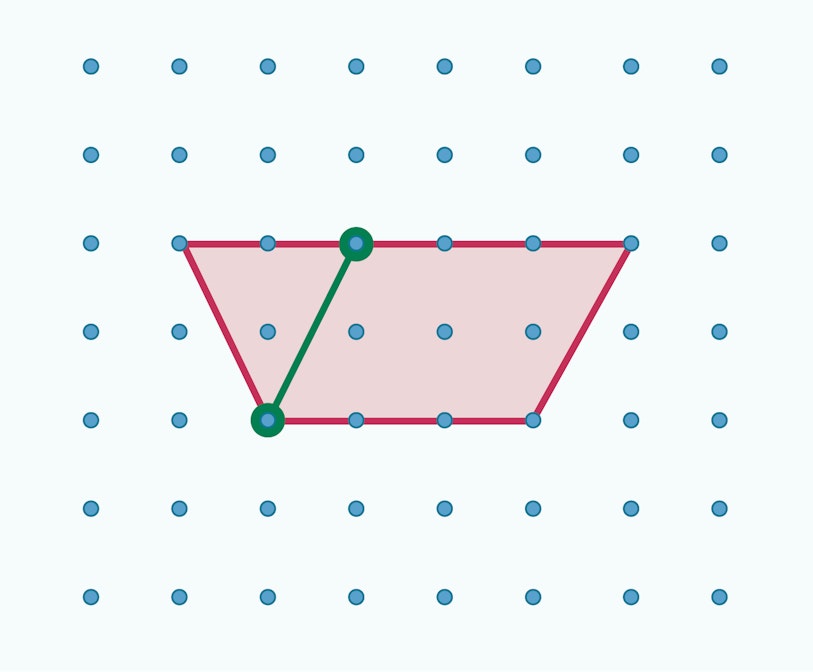
• Making a parallelogram inside the given trapezium using three of the sides.

• Drawing a diagonal to form two triangles.

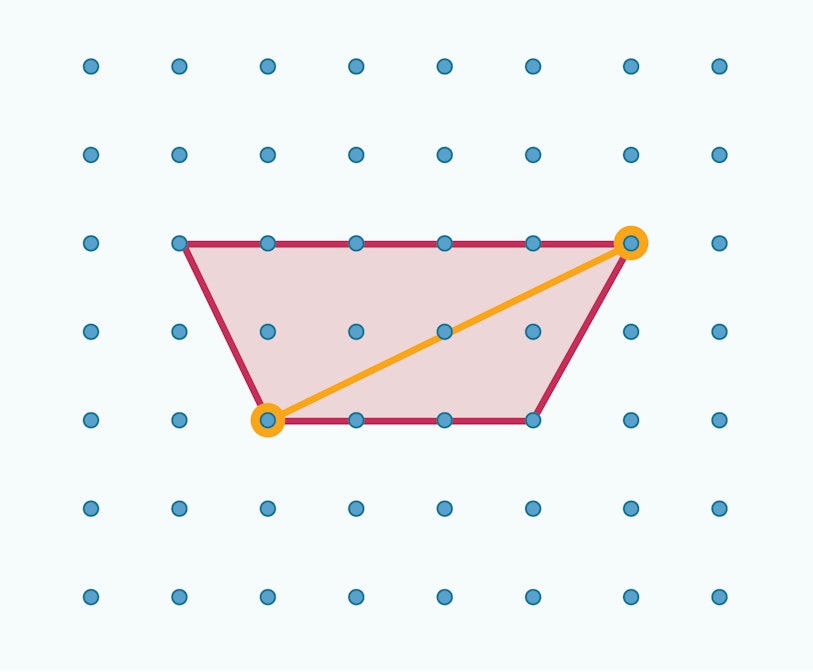
• Drawing a rectangle inside the trapezium, leaving two triangles, then putting those two triangles together.

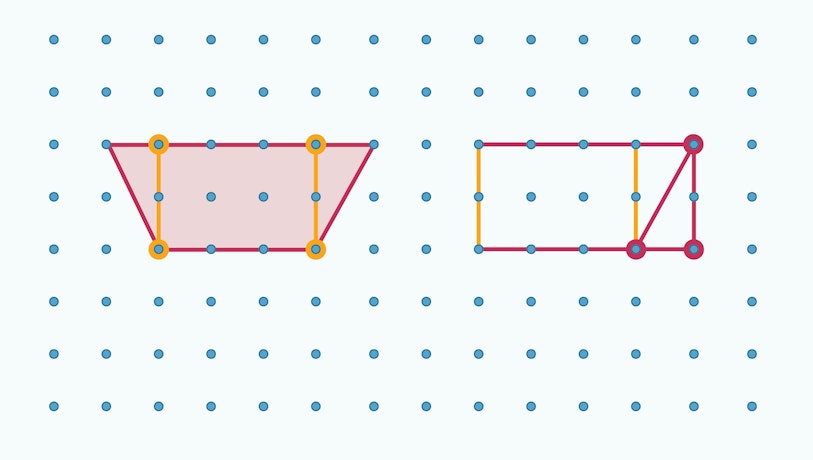
Encourage each group of students share and compare their methods with another group.

Challenge students to develop a formula for the area of trapeziums. Ask questions to prompt students to think about how they could use their prior knowledge about the area of parallelograms to help them develop the area formula for trapeziums, such as:

• How is a trapezium like a parallelogram?
• How can a trapezium be transformed to become a parallelogram?
• What do you notice about the area of a trapezium and the area of a parallelogram?
• Is there a rule that you could use to describe a way to work out the area of parallelograms? Could you explain your rule in words using the words ‘base’ and ‘height’? Could you explain your rule using mathematical symbols (formula)? A Frayer Model may be used to represent the formula in words and in symbols.

Advise students that for every hypothesis, mathematicians test their conjectures to ensure they are true for every type of trapeziums. Encourage students to consider if their derived formula is true for:

• trapeziums of different sizes
• trapeziums with different angles of slant.

Enable students requiring further support by modelling how to draw the trapeziums on grid paper and then physically cut out the shapes and transform them.

Extend students by challenging them to draw the trapeziums on plain paper, labelling the dimensions.

Areas for further exploration

Properties of quadrilaterals

The study of area of shapes complements the establishment of the properties of quadrilaterals. The Mathematics Curriculum Companion outlines the teaching context and suggests teaching ideas for this topic. 

Formative assessment of student learning in this stage of the sequence could include observation of students’ approaches as they discover how trapeziums are related to parallelograms as well as noting how students proceed to develop their formulas for finding the area of trapeziums.

Exit Tickets can be used to gather evidence to inform students’ understanding of the area formula. Invite students to reflect on their learning or to explain how the area formula for trapeziums is formulated. Alternatively, ask students to draw two different trapeziums and find their areas.

Government of South Australia, Department for Education and Child Development, 2017. Using units of measurement: Year 8. [Online]
Available at: https://acleadersresource.sa.edu.au/wp-content/uploads/2018/05/Using_units_of_measurement_Year_8.pdf
[Accessed 15 March 2022].

Math Learning Center, n.d. Geoboard. [Online]
Available at: https://apps.mathlearningcenter.org/geoboard/
[Accessed 15 March 2022].

Reys, R. E. et al., 2020. Helping Children Learn Mathematics. Milton: John Wiley & Sons Australia..

Siemon, D. et al., 2015. Teaching Mathematics: Foundations to Middle Years. Melbourne: Oxford University Press.

State Government of Victoria (Department of Education and Training), 2019. Introducing New Mathematical Terminology. [Online]
Available at: https://www.education.vic.gov.au/school/teachers/teachingresources/discipline/english/literacy/Pages/lim_terminology.aspx
[Accessed 15 March 2022].

State Government of Victoria (Department of Education and Training), 2020. Establish Properties of Quadrilaterals and Solve Related Problems Using Reasoning. [Online]
Available at: https://fuse.education.vic.gov.au/MCC/CurriculumItem?code=VCMMG293
[Accessed 15 March 2022].

The Teacher Toolkit, n.d. Exit Ticket. [Online]
Available at: https://www.theteachertoolkit.com/index.php/tool/exit-ticket
[Accessed 15 March 2022].

Van de Walle, J., Karp, K., M, B.-W. J. & Brass, A., 2019. Primary and Middle Years Mathematics: Teaching Developmentally. Australia: Pearson.