4. Operation Overload

• Operation overload 0 to 100 sheet: docx PDF 
• large classroom copy of the chart

This stage has been inspired by the Birth Year Puzzle problem on Maths300, and is reproduced with permission. Access to the problem on Maths300 requires a subscription.

The challenge in this task is to use four digits in a set order, with any legitimate mathematical operation, to create an expression equal to as many of the numbers from 0 to 100 as possible.

This task is like the relatively well known ‘Four 4s’ problem and can be applied using the birth year of the student. This has proven more problematic since the year 2000! Using a range of digits adds significant flexibility and extension opportunities.

You could select four digits from a year of local relevance to your students – the year that your school was opened, for example, or the year of birth of a significant local identity, or pick a random date, for example, December 18 could be turned into 1812. For the purposes of illustrating this task, the digits from the year 1968 will be used, in that order. 

Offer every student a 0 to 100 chart and use a large 0 to 100 wall chart to demonstrate.

Explain the task to students. Emphasise that the chosen digits must be used in the chosen order.

Invite contributions from the class, or show a few examples, to establish the ’rules’. Encourage students to use a variety of operations. For example:

• 19 + 6 - 8 = 17
• (1 + 9) x 6 + 8 = 68
• 1 + 9 - 6 + 8 = 12
• (-1 + 9) x 6 - 8 = 40

Model how to record the expression onto the 0 to 100 chart under the answer.

Provide students some time to start exploring the problem and create some of their own expressions for the shared class example.

Invite students to share their ‘creations’ and encourage them – after checking for accuracy – to enter them on the wall chart. By annotating these with a name or initials you can help give the students ownership of their work and help provide motivation for students to persist with the task.

Pose the challenge to the class:

‘Is it possible for us to completely fill up this wall chart? Can we create enough expressions to equal all the numbers from 0 to 100’?

Invite students to begin creating expressions and to add them to the class wall chart. You may like to establish some guidelines, such as asking students to check the accuracy of their expression with at least one other student before ‘publishing’. It is also a good idea to provide enough space on the wall chart for more than one expression for any given number to be entered.

Encourage students to move around and to collaborate on this task. Many small but useful discussions can flourish in this environment. Creating an environment where students are regularly exposed to the work of others (either through the checking process or the class wall chart) is fundamental for the learning opportunities that this task presents. Encourage students to move around and to collaborate on this task.

Depending on their previous exposure and confidence, students will select operations with which they feel comfortable working. Some will begin with combinations of basic operations (add, subtract, multiply, divide) which will allow them to have success and have access to the class wall chart. One of the key features of this task is its capacity to provide opportunities for success for students working at a variety of levels. Other students may be confident working with brackets, square roots, negative numbers and factorials.

For example, one student (say, Sunil) may create this expression:

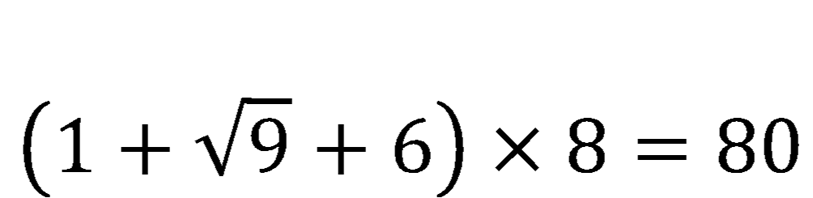
As you notice operations being used that you suspect are unfamiliar to your class, draw attention to them, and offer a short explicit teaching ‘mini lesson’ to students.

“Have a look at how Sunil created an expression equal to 80. If you’re familiar with the symbol on the 9 and what it means, Sunil’s expression might give you some good ideas for how to create some new expressions. If you’re not familiar with it, gather over here for a few minutes and I’ll explain it to you.”

In this way, students familiar with the concept of square roots are free to keep working, and only the students who need to be in the mini lesson are there. Additionally, their motivation to learn can be powerful, as you are offering them a new tool in their repertoire to create more expressions.

Look for opportunities to offer similar focussed mini lessons for the use of negative numbers, powers and factorials. If students do not generate expressions using these operations, present your own to the class and then offer a mini lesson to reinforce any particular concepts you feel appropriate for your students.

The motivation to complete the challenge can create a readiness in students to learn a new (and often quite advanced) operation, such as factorials. Ideally, these concepts would be raised by students, and you could ‘springboard’ off their ideas, but you can also introduce them, as well as some creativity, in this way: 

• Teacher: “Let me show you something called a factorial, which is written like this: 3!, and represents 3×2×1. So 3! is equal to 6. What would ‘four factorial’, or 4!, be equal to?
• Student: “4! would be equal to 4×3×2×1, which is equal to 24… but we don’t have a 4 in our numbers!”
• Teacher: “Can we use our numbers to make ‘four factorial’? For example: (1+the square root of 9)!”

There are explicit problem-solving strategies that can be addressed using this task. The following vignettes provide samples of how this could be done with students. They help illustrate the creativity that a mathematician can bring to their work.

1. Break a problem into manageable parts / Working backwards from the answer

A particular class noticed that the number 14 hadn’t been created. They decided to try and work backwards from 14 to find an expression.

The class noted that 6 + 8 = 14, but they still had to use the 1 and the 9.

Another student who was comfortable working with powers noted that 1 to the power of 9 equals 1, and that multiplying this by the 14, they would still have an expression equal to 14.

Hence:

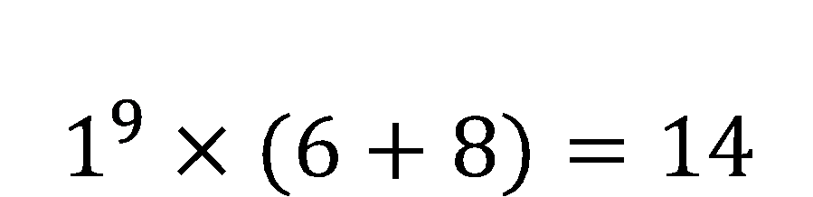
It is worth encouraging students to ‘think aloud’ with fellow students as they ‘hunt down’ missing answers.

2. Look for patterns

With these digits (1, 9, 6 and 8), a student recognised that once you can make a number using the 9, 6 and 8, you can then use the 1 to make a set of three consecutive numbers.

For example, 96 - 8 = 88.

Hence, three expressions can be made:

-1 + (96 - 8) = 87

1 X (96 - 8) = 88

1 + (96 - 8) = 89

This can motivate students to explore various combinations using only the 9, 6 and 8.

Facilitate a class discussion whereby students can collaboratively list and describe the various mathematical operations and techniques they have been exposed to. This will help them recognise the breadth of strategies available to them when working with number.

Consider setting these sample tasks for your students as a means of assessing their understanding of mathematical operators. These are specifically designed to help promote student agency.

• From the expressions you created, which one do you feel was the most complex, or sophisticated, and why? Which one are you most proud of?
• From the expressions your classmates created, which ones did you like, and why?
• What is a new mathematical operation you have learnt about by doing this task? Can you provide your own examples to show how this operation is used?
• Which numbers were you, or the class, unable to write an expression for? Why do you think this was the case?

Australian Association of Mathematics Teachers, n.d. Birth Year Puzzle. [Online]
Available at: maths300.com/members/m300full/177lyear.htm
[Accessed 15 March 2022; Note: access to this resource requires a maths300 subscription].