Suggested Learning Intentions
- To understand that a pattern is a predictable arrangement of elements
- To understand that patterns are determined by the application of different functions
- To understand the difference between repeated and growing patterns
Sample Success Criteria
- I can identify and describe a pattern
- I can model a pattern using a range of different manipulatives
- I can continue a pattern using appropriate functions (operations)
- I can create a pattern using a variety of functions (operations)
- I can articulate what a repeated pattern is and what a growing pattern is
Got it
This activity support students to notice and describe number patterns.
To begin, set students up in pairs with an interactive device between them. Ask students to play the game 'Got It'
Got It is an adding game for two players. The game starts with the target of 23. The first player selects an integer from 1 to 4. Players take turns to add an integer from 1 to 4 adding to the running total each time. The player who reaches 23 wins.
Encourage the students to play the game several times before asking them to reflect on a winning strategy. To support the development of a strategy, ask students to record each game.
Ask the following questions to support students’ understanding:
Can you tell who will win before the game reaches 23?
Lead students to see that if they reach 18 their partner can only add a maximum of 4 therefore leaving them to counter with the appropriate number to reach 23. Explore this further.
Ask: If you must reach 18 is there another number prior to this that you must reach? Can you identify all the key numbers to reach to ensure a winning strategy?
Encourage students to work backwards to identify all the key numbers.
Ask: Is there a counter strategy? If we were to change the winning number to 31 what would happen to these numbers? Did you notice a pattern? If so, can you describe it?
1. Garden Fences
This activity supports students to develop their ability to notice and describe patterns and rules, and to identify whether they are repeating or growing patterns. Students work towards formalising their understanding of patterns and rules by collecting and tabulating information and then describing the relationship between the number of fence posts and the length of the fence. Students compare the generalisation for each fence pattern and determine which one can be built based on the availability of materials.
Begin by asking the students if they know the difference between repeated and growing patterns.
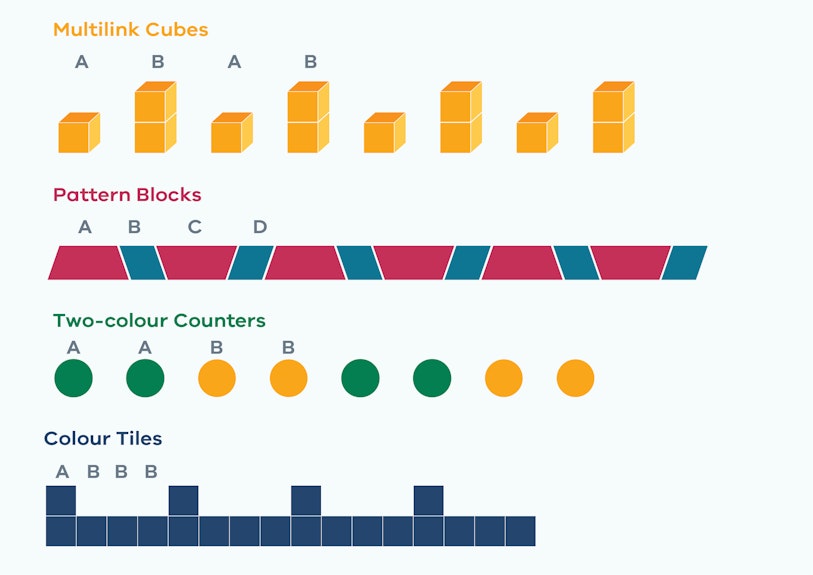
Repeating Pattern: a pattern where a core is identified and repeated multiple times. The unit of repeat is fixed. For example, red, blue, green, red, blue, green or 3, 2, 4, 3, 2, 4 (same 3 elements each time). See the image below for some examples.
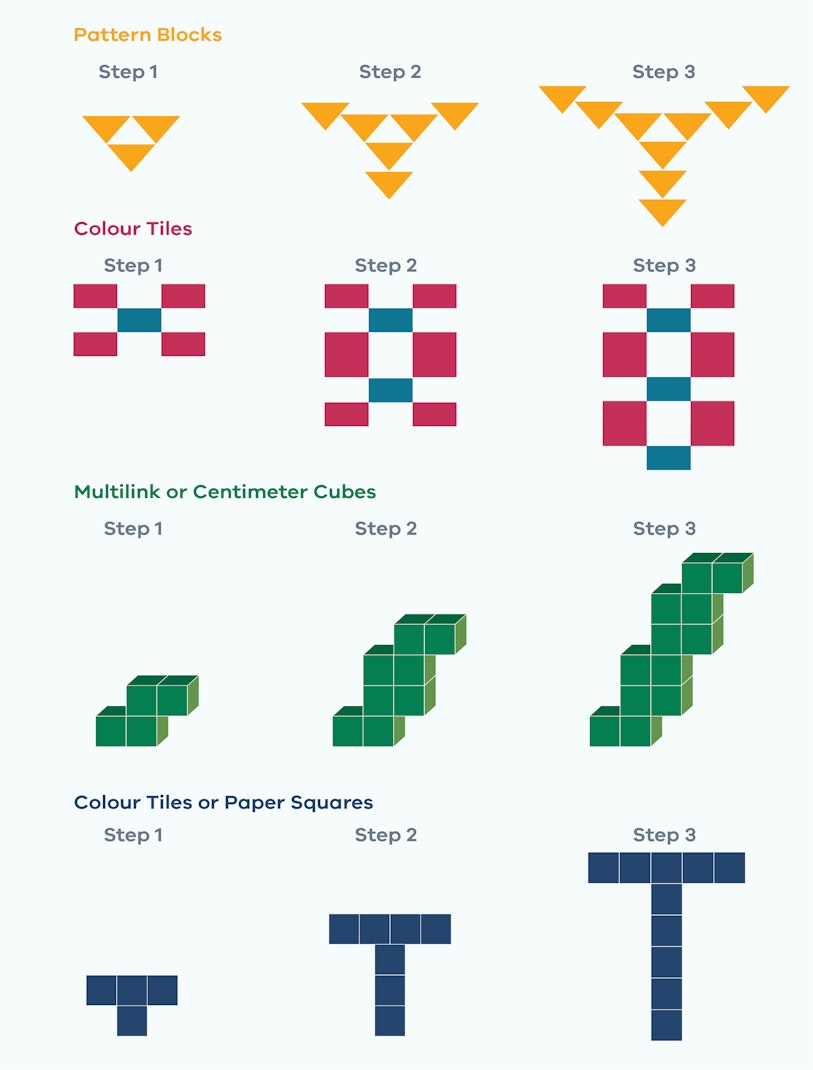
Growing Pattern: a pattern that involves a progression from step to step. It is where something is added every time the sequence repeats. For example, 2, 6, 12, 20 (+4, +6, +8). See the image below for some examples.
Provide students with the following scenario:
Hanh works on a farm, and he is in charge of building fences which prevent the animals from escaping their paddocks. He can build the fences using two different patterns. He picks the pattern based on the number of wooden posts he has available, and the length of the fence needed. Hanh is wondering if it is possible to come up with a pattern which allows him to calculate how many wooden posts are needed for each pattern. Each post is one metre in length.
See the image below for the patterns. Each fence starts at a brick wall, which is already in place at the farm.

Encourage students to work in pairs to draw or build (using pop sticks) the first six terms of each pattern and then tabulate their findings in the table below. Physically building the pattern supports students to identify the repeating components of a pattern.
Fence Pattern 1
| Metres | 1 | 2 | 3 | 4 | 5 | 6 |
| Posts |
Fence Pattern 2
| Metres | 1 | 2 | 3 | 4 | 5 | 6 |
| Posts |
Using the information contained within both tables ask students to describe the pattern using words and numbers. Ask students to explain whether the pattern is a repeating pattern or a growing pattern and explain how they know.
Enable students by providing them with pop sticks to build each fence. Encourage students to extend the table and add each new length into their tables. Ask them to talk aloud about what they are doing each time they add a new length of fence. Ask: ‘How many pop sticks do you add each time?’ Encourage students to make a generalisation and define the rule by guiding them with the following questions:
- How many sticks do you keep adding to the fence to make the next length?
- What pattern do you see?
Extend students by guiding them to formalise the relationship in an algebraic expression. Ask the following questions:
- Can you see the pattern?
- How did you work that out?
- What would you do if you needed to build a 20-metre fence? Would you just keep adding 2? Or could you do it a quicker way? How many sticks do you need for each metre length?
Now ask the students to have a go at writing the rules in an algebraic equation. (Answer: y = 2x and y = 3x.) Ask students to use their generalisation to determine how many posts Hanh would need to make a 10-metre long fence for each pattern.
You can also extend students in a slightly different direction by asking them to determine how big a fence Hanh could build if he had 24 fence posts. (Answer: he could build a 12-metre fence or an 8-metre fence.) Ask, is there a number less than 24 in which Hanh could build either fence pattern and have no left over posts? (Answer: 6) Demonstrate that this is because both 2 and 3 are factors of 6 and 24.
2. Four-Arm Shapes
This lesson supports students to develop their pattern making skills and their ability to describe patterns and rules. Students will work towards formalising their understanding of patterns and rules by collecting and tabulating information and then describing the relationship between the number of tiles and the length of the arms in the shapes.
Encourage students to draw or build (using counters) the first six terms in the pattern below.
Provide students with the following scenario:
A council gardener is designing a new pathway for people to walk on in a local park. She wants to build four equal paths using square tiles that will all meet ‘in the middle’. She calls this a ‘four-arm path’ as it forms a cross.

The council gardener begins thinking about how she might build additional pathways similar to this in a range of parks within the municipality. Some of the parks are quite large, while others are much smaller. She begins to wonder if it is possible to come up with a pattern which allows her to calculate how many tiles are needed for each size.
Encourage students to work in pairs to calculate how many tiles are needed to make the first 6 terms. Ask them to place their information in a table.
| Arm length | 1 | 2 | 3 | 4 | 5 | 6 |
| Number of tiles | 5 |
Using the information contained within the table, ask students to describe the pattern using words and numbers. For example, ‘For every arm after the first one, you need to add four more tiles (arm length x 4 + 1).
Enable students by guiding them to define the rule and to make generalisations with targeted questioning. See below for an example:
- How many tiles in total did you need to make an arm length of two?
- How many tiles in total for an arm length of three then?
- Can you write instructions for another student on how to build the pattern?
Extend students by guiding them to formalise the relationship into an algebraic expression. Ask the following questions:
- How many tiles do you need each time you add an arm length?
- How many tiles do you start with before adding any arms?
- Write the rule now, using an algebraic equation. (Answer: y = 4x + 1)
Now ask students to use their generalisation to determine how many tiles the gardener would need to make a path that has an arm length of 10.
Enable students by providing them with counters to build the path. Ask: "Do you need to build each arm of the path? If you just built one, could you work out the answer?"
Extend students by asking them to calculate how many tiles are needed for a path which reaches 80 tiles in each direction. (The deliberate choice of such a large number, 80, forces students to use the rule as opposed to building the path with 321 tiles).
Areas for further exploration
1. Pattern recognition and building
Take a class tour of the school yard or local environment and have students point out patterns that they see. Some examples might be:
- A repeating pattern of tiles.
- Tessellated tiles
- The number sequence of house numbers
- A staircase
Arrange several pattern stations around your classroom. Students form small groups and move from station to station. Students record the pattern in their workbooks and determine the next three elements in the pattern. Ask students to describe the pattern using words and symbols.
Consider a range of patterns:
- Simple repeating patterns (red, blue, green, red, blue, green, red, blue, green)
- Number sequences (1, 2, 3, 1, 2, 3 or 4, 8, 12, 16)
- Triangular numbers (1, 3, 6, 10, 15, 21)
- Growing patterns (red; red, blue; red, blue, green; red, blue, green, yellow)
Enable students to recognise patterns by first providing them with a pattern and then ask them to copy the pattern and then continue the pattern. Encourage students to describe aloud how each pattern works.
Extend students by asking them to come up with their own pattern for each of the types of patterns listed above (simple repeating, number sequences, etc).
To further consolidate and assess students’ understanding encourage them to apply the knowledge gained from the Four-Arm Shape activity (the relationship between the number of the tiles per arm and the total number of tiles required to build path). Have students work backwards to determine the number of tiles of each arm’s length if they know the total number of tiles used in the path:
If the gardener took 85 tiles with her to a job, how big a design could she make? Explain your working.
Enable students by reducing the total number of tiles used to 33. Provide them with 33 tiles to allow them to build the path.
Extend students by providing them with the following enhanced scenario:
The gardener records how many tiles she uses for each design. Last week, she built 5 different ‘four-arm’ paths in the park. She recorded her results in this table, but she thinks that she recorded some numbers incorrectly. Which ones do you think are incorrect, and why?
| Day the path was built | Monday | Tuesday | Wednesday | Thursday | Friday |
| Tiles used | 65 | 101 | 19 | 201 | 132 |
Encourage students to reflect on their ability to describe a pattern. Some students will only be able to describe a pattern using words while others will be able to use algebra to describe a pattern. The following may help you to assess student understanding. Can the student:
- describe the pattern using words and maths?
- describe, create, and continue a variety of patterns?
- identify whether a pattern is repeating or growing?
- describe the rule used to create the sequence/pattern?
Australian Academy of Science, n.d. Algebra: Equivalence. [Online]
Available at: https://www.resolve.edu.au/algebra-equivalence
[Accessed 15 March 2022].
Australian Association of Mathematics Teachers, n.d. 4 Arm Shapes. [Online]
Available at: maths300.com/members/m300full/040l4arm.htm
[Accessed 15 March 2022; Note: access to this resource requires a maths300 subscription].
Melbourne Archdiocese Catholic Schools, 2013. Key Ideas for Concept Development in Mathematics. East Melbourne: Melbourne Archdiocese Catholic Schools.
Nathan, M. & Knuth, E., 2003. A study of whole classroom mathematical discourse and teacher change. Cognition and instruction, 21(2), pp. 175-207.
Parsons, J. & Reilly, Y., 2012. Meet the Johnsons. Maths in the Inclusive Classroom Book 1. s.l.:Essential Resources.
Philips, E., Gardella, T., Kelly, C. & Stewart, J., 1991. Patterns and Functions, Addenda Series, Grades 5-6. Reston, VA: NCTM.
Siemon, D. et al., 2015. Teaching Mathematics: Foundations to Middle Years. Melbourne: Oxford University Press.
State Government of Victoria, (Department of Education and Training), 2017. Monster choir: making patterns. [Online]
Available at: https://fuse.education.vic.gov.au/Resource/LandingPage?ObjectId=e066b087-7026-479e-9f9e-7b1f4b0422c1&SearchScope=Primary
[Accessed 15 March 2022].
State Government of Victoria, (Department of Education and Training), n.d. Introduction of variables using letters to represent numbers. [Online]
Available at: https://fuse.education.vic.gov.au/mcc/CurriculumItem?code=VCMNA251
[Accessed 15 March 2022].
Stein, C., 2007. Promoting mathematical discourse in the classroom. NCTM, vol 101, no.4. [Online]
Available at: http://hybridalgebra.pbworks.com/f/lets+talk+promoting+mathematical+discourse+in+the+classroom.pdf
[Accessed 15 March 2022].
Sullivan, P., 2017. Challenging Mathematical Tasks. South Melbourne: Oxford University Press.
TopMarks, n.d. Function Machines. [Online]
Available at: https://www.topmarks.co.uk/Flash.aspx?f=FunctionMachinev3
[Accessed 15 March 2022].
Toy Theatre, 2001-2022. Number Pattern. [Online]
Available at: http://toytheater.com/number-pattern/
[Accessed 15 March 2022].
University of Cambridge, n.d. NRICH: Got It. [Online]
Available at: https://nrich.maths.org/1272/index
[Accessed 15 March 2022].
University of Cambridge, n.d. NRICH: Mathematics Resources for Teachers, Parents and Children. [Online]
Available at: https://nrich.maths.org/
[Accessed 15 March 2022].
University of Cambridge, n.d. NRICH: Sticky Triangles. [Online]
Available at: https://nrich.maths.org/88/index
[Accessed 15 March 2022].
Uttal, D., Scudder, K. & DeLoache, J., 1997. Manipulatives as symbols: A new perspective on the use of concrete objects to teach mathematics. Journal of applied developmental psychology, 18(1), pp. 37-54.
Van de Walle, J., Karp, K., M, B.-W. J. & Brass, A., 2019. Primary and Middle Years Mathematics: Teaching Developmentally. Australia: Pearson.
Other stages
1. Functions and Relations
EXPLORESuggested Learning Intentions
- To understand that functions describe the relationships between numbers
- To understand that a relation is a set of inputs and outputs
- To understand that a function is a relation with one output for each input
Sample Success Criteria
- I can explain that the relationship between one number and another number is described by the functions performed on them
- I can articulate that a relation is a set of inputs and outputs
- I can determine the output of a number given a known input
- I can demonstrate the effect of a function being performed using a variety of manipulatives
3. Equivalence
EXPLORESuggested Learning Intentions
- To understand that expressions on either side of an equal sign must be balanced
- To explain that when a function on one side of an equation is performed an equal function must be performed on the other side to maintain balance
Sample Success Criteria
- I can articulate that an equals sign represents a balance of the expressions on either side of it
- I can create equivalent number sentences/equations
- I can manipulate equations or number sentences to maintain the balance on both sides
- I can model my problem solutions using a variety of manipulatives
4. Using Symbols to Recognise Unknowns
EXPLORESuggested Learning Intentions
- To understand that symbols (pronumerals) can be used to represent a variable or an unknown quantity in an equation
- To understand that symbols can be used to represent an unknown in a pattern or a generalisation
- To understand that these equations can be manipulated to determine the unknown quantity
Sample Success Criteria
- I can use a symbol (pronumeral) to represent an unknown in an equation
- I can use a symbol (pronumeral) to represent an unknown in a pattern
- I can manipulate equations to determine the unknown (symbol/pronumeral)
- I can justify my thinking using other representations of the mathematics